
Magnetic Prandtl number
Encyclopedia
The Magnetic Prandtl number is a dimensionless quantity
occurring in magnetohydrodynamics
which approximates the ratio of momentum diffusivity (viscosity
) and magnetic diffusivity. It is defined as:

where:
At the base of the Sun
's convection zone
the Magnetic Prandtl number is approximately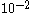 , and in the interiors of planets and in liquid-metal laboratory dynamos is approximately
, and in the interiors of planets and in liquid-metal laboratory dynamos is approximately 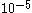 .
.
Dimensionless quantity
In dimensional analysis, a dimensionless quantity or quantity of dimension one is a quantity without an associated physical dimension. It is thus a "pure" number, and as such always has a dimension of 1. Dimensionless quantities are widely used in mathematics, physics, engineering, economics, and...
occurring in magnetohydrodynamics
Magnetohydrodynamics
Magnetohydrodynamics is an academic discipline which studies the dynamics of electrically conducting fluids. Examples of such fluids include plasmas, liquid metals, and salt water or electrolytes...
which approximates the ratio of momentum diffusivity (viscosity
Viscosity
Viscosity is a measure of the resistance of a fluid which is being deformed by either shear or tensile stress. In everyday terms , viscosity is "thickness" or "internal friction". Thus, water is "thin", having a lower viscosity, while honey is "thick", having a higher viscosity...
) and magnetic diffusivity. It is defined as:

where:
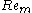 is the magnetic Reynolds numberMagnetic Reynolds numberThe Magnetic Reynolds number is a dimensionless group thatoccurs in magnetohydrodynamics. It gives an estimate of the effects of magnetic advection to magnetic diffusion, and is typically defined by:where* U is a typical velocity scale of the flow...
is the magnetic Reynolds numberMagnetic Reynolds numberThe Magnetic Reynolds number is a dimensionless group thatoccurs in magnetohydrodynamics. It gives an estimate of the effects of magnetic advection to magnetic diffusion, and is typically defined by:where* U is a typical velocity scale of the flow...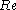 is the Reynolds number
is the Reynolds number is the momentum diffusivity (kinematic viscosity)
is the momentum diffusivity (kinematic viscosity) is the magnetic diffusivity
is the magnetic diffusivity
At the base of the Sun
Sun
The Sun is the star at the center of the Solar System. It is almost perfectly spherical and consists of hot plasma interwoven with magnetic fields...
's convection zone
Convection zone
The convection zone of a star is the range of radii in which energy is transported primarily by convection. In the radiation zone, energy is transported by radiation...
the Magnetic Prandtl number is approximately
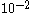 , and in the interiors of planets and in liquid-metal laboratory dynamos is approximately
, and in the interiors of planets and in liquid-metal laboratory dynamos is approximately 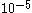 .
.

