
Hodge index theorem
Encyclopedia
In mathematics
, the Hodge index theorem for an algebraic surface
V determines the signature of the intersection pairing on the algebraic curve
s C on V. It says, roughly speaking, that the space spanned by such curves (up to linear equivalence) has a one-dimensional subspace on which it is positive definite (not uniquely determined), and decomposes as a direct sum
of some such one-dimensional subspace, and a complementary subspace on which it is negative definite.
In a more formal statement, specify that V is a non-singular projective variety, and let H be the divisor class on V of a hyperplane section
of V in a given projective embedding. Then the intersection
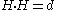
where d is the degree
of V (in that embedding). Let D be the vector space of rational divisor classes on V, up to algebraic equivalence. The dimension of D is finite and is usually denoted by ρ(V). The Hodge index theorem says that the subspace spanned by H in D has a complementary subspace on which the intersection pairing is negative definite. Therefore the signature (often also called index) is (1,ρ(V)).
The abelian group of divisor classes up to algebraic equivalence is now called the Néron-Severi group; it is known to be a finitely-generated abelian group, and the result is about its tensor product
with the rational number field. Therefore ρ(V) is equally the rank of the Néron-Severi group (which can have a non-trivial torsion subgroup
, on occasion).
This result was proved in the 1930s by W. V. D. Hodge
, for varieties over the complex numbers, after it had been a conjecture for some time of the Italian school of algebraic geometry
(in particular, Francesco Severi
, who in this case showed that ρ < ∞). Hodge's methods were the topological ones brought in by Lefschetz. The result holds over general (algebraically closed) fields.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, the Hodge index theorem for an algebraic surface
Algebraic surface
In mathematics, an algebraic surface is an algebraic variety of dimension two. In the case of geometry over the field of complex numbers, an algebraic surface has complex dimension two and so of dimension four as a smooth manifold.The theory of algebraic surfaces is much more complicated than that...
V determines the signature of the intersection pairing on the algebraic curve
Algebraic curve
In algebraic geometry, an algebraic curve is an algebraic variety of dimension one. The theory of these curves in general was quite fully developed in the nineteenth century, after many particular examples had been considered, starting with circles and other conic sections.- Plane algebraic curves...
s C on V. It says, roughly speaking, that the space spanned by such curves (up to linear equivalence) has a one-dimensional subspace on which it is positive definite (not uniquely determined), and decomposes as a direct sum
Direct sum of modules
In abstract algebra, the direct sum is a construction which combines several modules into a new, larger module. The result of the direct summation of modules is the "smallest general" module which contains the given modules as submodules...
of some such one-dimensional subspace, and a complementary subspace on which it is negative definite.
In a more formal statement, specify that V is a non-singular projective variety, and let H be the divisor class on V of a hyperplane section
Hyperplane section
In mathematics, a hyperplane section of a subset X of projective space Pn is the intersection of X with some hyperplane H — in other words we look at the subset XH of those elements x of X that satisfy the single linear condition L = 0 defining H as a linear subspace...
of V in a given projective embedding. Then the intersection
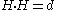
where d is the degree
Degree of an algebraic variety
The degree of an algebraic variety in mathematics is defined, for a projective variety V, by an elementary use of intersection theory.For V embedded in a projective space Pn and defined over some algebraically closed field K, the degree d of V is the number of points of intersection of V, defined...
of V (in that embedding). Let D be the vector space of rational divisor classes on V, up to algebraic equivalence. The dimension of D is finite and is usually denoted by ρ(V). The Hodge index theorem says that the subspace spanned by H in D has a complementary subspace on which the intersection pairing is negative definite. Therefore the signature (often also called index) is (1,ρ(V)).
The abelian group of divisor classes up to algebraic equivalence is now called the Néron-Severi group; it is known to be a finitely-generated abelian group, and the result is about its tensor product
Tensor product
In mathematics, the tensor product, denoted by ⊗, may be applied in different contexts to vectors, matrices, tensors, vector spaces, algebras, topological vector spaces, and modules, among many other structures or objects. In each case the significance of the symbol is the same: the most general...
with the rational number field. Therefore ρ(V) is equally the rank of the Néron-Severi group (which can have a non-trivial torsion subgroup
Torsion subgroup
In the theory of abelian groups, the torsion subgroup AT of an abelian group A is the subgroup of A consisting of all elements that have finite order...
, on occasion).
This result was proved in the 1930s by W. V. D. Hodge
W. V. D. Hodge
William Vallance Douglas Hodge FRS was a Scottish mathematician, specifically a geometer.His discovery of far-reaching topological relations between algebraic geometry and differential geometry—an area now called Hodge theory and pertaining more generally to Kähler manifolds—has been a major...
, for varieties over the complex numbers, after it had been a conjecture for some time of the Italian school of algebraic geometry
Italian school of algebraic geometry
In relation with the history of mathematics, the Italian school of algebraic geometry refers to the work over half a century or more done internationally in birational geometry, particularly on algebraic surfaces. There were in the region of 30 to 40 leading mathematicians who made major...
(in particular, Francesco Severi
Francesco Severi
Francesco Severi was an Italian mathematician.Severi was born in Arezzo, Italy. He is famous for his contributions to algebraic geometry and the theory of functions of several complex variables. He became the effective leader of the Italian school of algebraic geometry...
, who in this case showed that ρ < ∞). Hodge's methods were the topological ones brought in by Lefschetz. The result holds over general (algebraically closed) fields.

