
Hilbert–Poincaré series
Encyclopedia
In mathematics
, and in particular in the field of algebra
, a Hilbert–Poincaré series (also known under the name Hilbert series), named after David Hilbert
and Henri Poincaré
, is an adaptation of the notion of dimension
to the context of graded algebraic structures (where the dimension of the entire structure is often infinite). It is a formal power series in one indeterminate, say t, where the coefficient of tn gives the dimension (or rank) of the sub-structure of elements homogeneous of degree n. It is closely related to the Hilbert polynomial
in cases when the latter exists; however, the Hilbert–Poincaré series describes the rank in every degree, while the Hilbert polynomial describes in only in all but finitely many degrees, and therefore provides less information. In particular the Hilbert–Poincaré series cannot be deduced from the Hilbert polynomial even if the latter exists. In good cases, the Hilbert–Poincaré series can be expressed as a rational function
of its argument t.
 be a N-graded vector space
be a N-graded vector space
over K, where each subspace Vi of vectors of degree n is finite dimensional. Then the Hilbert–Poincaré series of V is the formal power series
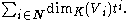
A similar definition can be given for an N-graded R-module over any commutative ring
R in which each submodule of elements homogeneous of a fixed degree n is free
of finite rank; it suffices to replace the dimension by the rank. Often the graded vector space or module of which the Hilbert–Poincaré series is considered has additional structure, for instance that of a ring, but the Hilbert–Poincaré series is independent of the multiplicative or other structure.
s (Xi)i∈N form a basis of the underlying vector space, formed of homogeneous elements, the dimension of each homogeneous component is 1, and the Hilbert–Poincaré series of K[X] is therefore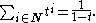
For a polynomial ring K[X,Y] in two indeterminates, graded by total degree, the monomials again form a vector space basis formed of homogeneous elements; this time there are d + 1 monomials of degree d for any d, namely Xd, Xd–1Y, Xd–2Y2, …, Yd. Therefore the Hilbert–Poincaré series of K[X,Y] is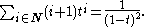
The fact that this is the square of the Hilbert–Poincaré series of K[X] is no accident: one has K[X,Y] = K[X] ⊗ K[Y] as graded rings, and in general the Hilbert–Poincaré series of the tensor product of graded vector spaces is the product of their Hilbert–Poincaré series. It follows that the Hilbert–Poincaré series of K[X1,X2,…,Xn] is
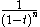
for any n.
, or cochain complex C of vector spaces; the latter takes the form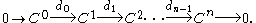
The Hilbert–Poincaré series (here often called the Poincaré polynomial) of the graded vector space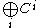 for this complex is
for this complex is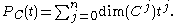
The Hilbert-Poincaré polynomial of the cohomology
, with cohomology spaces Hj = Hj(C), is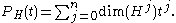
A famous relation between the two is that there is a polynomial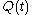 with non-negative coefficients, such that
with non-negative coefficients, such that

Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, and in particular in the field of algebra
Algebra
Algebra is the branch of mathematics concerning the study of the rules of operations and relations, and the constructions and concepts arising from them, including terms, polynomials, equations and algebraic structures...
, a Hilbert–Poincaré series (also known under the name Hilbert series), named after David Hilbert
David Hilbert
David Hilbert was a German mathematician. He is recognized as one of the most influential and universal mathematicians of the 19th and early 20th centuries. Hilbert discovered and developed a broad range of fundamental ideas in many areas, including invariant theory and the axiomatization of...
and Henri Poincaré
Henri Poincaré
Jules Henri Poincaré was a French mathematician, theoretical physicist, engineer, and a philosopher of science...
, is an adaptation of the notion of dimension
Dimension
In physics and mathematics, the dimension of a space or object is informally defined as the minimum number of coordinates needed to specify any point within it. Thus a line has a dimension of one because only one coordinate is needed to specify a point on it...
to the context of graded algebraic structures (where the dimension of the entire structure is often infinite). It is a formal power series in one indeterminate, say t, where the coefficient of tn gives the dimension (or rank) of the sub-structure of elements homogeneous of degree n. It is closely related to the Hilbert polynomial
Hilbert polynomial
In commutative algebra, the Hilbert polynomial of a graded commutative algebra or graded module is a polynomial in one variable that measures the rate of growth of the dimensions of its homogeneous components...
in cases when the latter exists; however, the Hilbert–Poincaré series describes the rank in every degree, while the Hilbert polynomial describes in only in all but finitely many degrees, and therefore provides less information. In particular the Hilbert–Poincaré series cannot be deduced from the Hilbert polynomial even if the latter exists. In good cases, the Hilbert–Poincaré series can be expressed as a rational function
Rational function
In mathematics, a rational function is any function which can be written as the ratio of two polynomial functions. Neither the coefficients of the polynomials nor the values taken by the function are necessarily rational.-Definitions:...
of its argument t.
Definition
Let K be a field, and let be a N-graded vector space
be a N-graded vector spaceGraded vector space
In mathematics, a graded vector space is a type of vector space that includes the extra structure of gradation, which is a decomposition of the vector space into a direct sum of vector subspaces.-N-graded vector spaces:...
over K, where each subspace Vi of vectors of degree n is finite dimensional. Then the Hilbert–Poincaré series of V is the formal power series
Formal power series
In mathematics, formal power series are a generalization of polynomials as formal objects, where the number of terms is allowed to be infinite; this implies giving up the possibility to substitute arbitrary values for indeterminates...
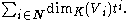
A similar definition can be given for an N-graded R-module over any commutative ring
Commutative ring
In ring theory, a branch of abstract algebra, a commutative ring is a ring in which the multiplication operation is commutative. The study of commutative rings is called commutative algebra....
R in which each submodule of elements homogeneous of a fixed degree n is free
Free module
In mathematics, a free module is a free object in a category of modules. Given a set S, a free module on S is a free module with basis S.Every vector space is free, and the free vector space on a set is a special case of a free module on a set.-Definition:...
of finite rank; it suffices to replace the dimension by the rank. Often the graded vector space or module of which the Hilbert–Poincaré series is considered has additional structure, for instance that of a ring, but the Hilbert–Poincaré series is independent of the multiplicative or other structure.
Examples
A basic example of a graded vector space is the polynomial ring K[X], graded by degree. Since the monomialMonomial
In mathematics, in the context of polynomials, the word monomial can have one of two different meanings:*The first is a product of powers of variables, or formally any value obtained by finitely many multiplications of a variable. If only a single variable x is considered, this means that any...
s (Xi)i∈N form a basis of the underlying vector space, formed of homogeneous elements, the dimension of each homogeneous component is 1, and the Hilbert–Poincaré series of K[X] is therefore
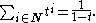
For a polynomial ring K[X,Y] in two indeterminates, graded by total degree, the monomials again form a vector space basis formed of homogeneous elements; this time there are d + 1 monomials of degree d for any d, namely Xd, Xd–1Y, Xd–2Y2, …, Yd. Therefore the Hilbert–Poincaré series of K[X,Y] is
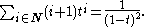
The fact that this is the square of the Hilbert–Poincaré series of K[X] is no accident: one has K[X,Y] = K[X] ⊗ K[Y] as graded rings, and in general the Hilbert–Poincaré series of the tensor product of graded vector spaces is the product of their Hilbert–Poincaré series. It follows that the Hilbert–Poincaré series of K[X1,X2,…,Xn] is
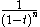
for any n.
Chain complex
An example of graded vector space is associated to a chain complexChain complex
In mathematics, chain complex and cochain complex are constructs originally used in the field of algebraic topology. They are algebraic means of representing the relationships between the cycles and boundaries in various dimensions of some "space". Here the "space" could be a topological space or...
, or cochain complex C of vector spaces; the latter takes the form
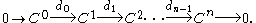
The Hilbert–Poincaré series (here often called the Poincaré polynomial) of the graded vector space
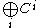 for this complex is
for this complex is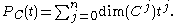
The Hilbert-Poincaré polynomial of the cohomology
Cohomology
In mathematics, specifically in algebraic topology, cohomology is a general term for a sequence of abelian groups defined from a co-chain complex. That is, cohomology is defined as the abstract study of cochains, cocycles, and coboundaries...
, with cohomology spaces Hj = Hj(C), is
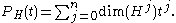
A famous relation between the two is that there is a polynomial
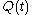 with non-negative coefficients, such that
with non-negative coefficients, such that

