
Heptapeton
Encyclopedia
In geometry
, a 6-simplex
is a self-dual regular
6-polytope
. It has 7 vertices
, 21 edge
s, 35 triangle faces
, 35 tetrahedral
cells, 21 5-cell 4-faces, and 7 5-simplex 5-faces. Its dihedral angle
is cos−1(1/6), or approximately 80.41°.
in Greek
and -peta for having five-dimensional facets, and -on. Jonathan Bowers gives a heptapeton the acronym hop.
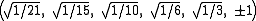
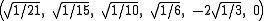
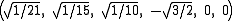

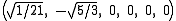
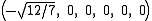
The vertices of the 6-simplex can be more simply positioned in 7-space as permutations of:
This construction is based on facets of the 7-orthoplex.
, all shown here in A6 Coxeter plane orthographic projection
s.
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
, a 6-simplex
Simplex
In geometry, a simplex is a generalization of the notion of a triangle or tetrahedron to arbitrary dimension. Specifically, an n-simplex is an n-dimensional polytope which is the convex hull of its n + 1 vertices. For example, a 2-simplex is a triangle, a 3-simplex is a tetrahedron,...
is a self-dual regular
Regular polytope
In mathematics, a regular polytope is a polytope whose symmetry is transitive on its flags, thus giving it the highest degree of symmetry. All its elements or j-faces — cells, faces and so on — are also transitive on the symmetries of the polytope, and are regular polytopes of...
6-polytope
6-polytope
In six-dimensional geometry, a uniform polypeton is a six-dimensional uniform polytope. A uniform polypeton is vertex-transitive, and all facets are uniform polytera....
. It has 7 vertices
Vertex (geometry)
In geometry, a vertex is a special kind of point that describes the corners or intersections of geometric shapes.-Of an angle:...
, 21 edge
Edge (geometry)
In geometry, an edge is a one-dimensional line segment joining two adjacent zero-dimensional vertices in a polygon. Thus applied, an edge is a connector for a one-dimensional line segment and two zero-dimensional objects....
s, 35 triangle faces
Face (geometry)
In geometry, a face of a polyhedron is any of the polygons that make up its boundaries. For example, any of the squares that bound a cube is a face of the cube...
, 35 tetrahedral
Tetrahedron
In geometry, a tetrahedron is a polyhedron composed of four triangular faces, three of which meet at each vertex. A regular tetrahedron is one in which the four triangles are regular, or "equilateral", and is one of the Platonic solids...
cells, 21 5-cell 4-faces, and 7 5-simplex 5-faces. Its dihedral angle
Dihedral angle
In geometry, a dihedral or torsion angle is the angle between two planes.The dihedral angle of two planes can be seen by looking at the planes "edge on", i.e., along their line of intersection...
is cos−1(1/6), or approximately 80.41°.
Alternate names
It can also be called a heptapeton, or hepta-6-tope, as a 7-facetted polytope in 6-dimensions. The name heptapeton is derived from hepta for seven facetsFacet (mathematics)
A facet of a simplicial complex is a maximal simplex.In the general theory of polyhedra and polytopes, two conflicting meanings are currently jostling for acceptability:...
in Greek
Greek language
Greek is an independent branch of the Indo-European family of languages. Native to the southern Balkans, it has the longest documented history of any Indo-European language, spanning 34 centuries of written records. Its writing system has been the Greek alphabet for the majority of its history;...
and -peta for having five-dimensional facets, and -on. Jonathan Bowers gives a heptapeton the acronym hop.
Coordinates
The Cartesian coordinates for an origin-centered regular heptapeton having edge length 2 are: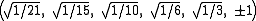
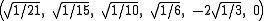
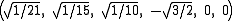

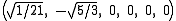
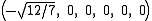
The vertices of the 6-simplex can be more simply positioned in 7-space as permutations of:
- (0,0,0,0,0,0,1)
This construction is based on facets of the 7-orthoplex.
Related uniform 6-polytopes
The regular 6-simplex is one of 35 uniform 6-polytopes based on the [3,3,3,3,3] Coxeter groupCoxeter group
In mathematics, a Coxeter group, named after H.S.M. Coxeter, is an abstract group that admits a formal description in terms of mirror symmetries. Indeed, the finite Coxeter groups are precisely the finite Euclidean reflection groups; the symmetry groups of regular polyhedra are an example...
, all shown here in A6 Coxeter plane orthographic projection
Orthographic projection
Orthographic projection is a means of representing a three-dimensional object in two dimensions. It is a form of parallel projection, where all the projection lines are orthogonal to the projection plane, resulting in every plane of the scene appearing in affine transformation on the viewing surface...
s.

