
Harish-Chandra transform
Encyclopedia
In mathematical representation theory
, the Harish-Chandra transform is a linear map from functions on a reductive Lie group to functions on a parabolic subgroup. It was introduced by .
The Harish-Chandra transform fP of a function f on the group G is given by
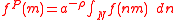
where P = MAN is the Langlands decomposition
of a parabolic subgroup.
Representation theory
Representation theory is a branch of mathematics that studies abstract algebraic structures by representing their elements as linear transformations of vector spaces, and studiesmodules over these abstract algebraic structures...
, the Harish-Chandra transform is a linear map from functions on a reductive Lie group to functions on a parabolic subgroup. It was introduced by .
The Harish-Chandra transform fP of a function f on the group G is given by
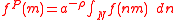
where P = MAN is the Langlands decomposition
Langlands decomposition
In mathematics, the Langlands decomposition writes a parabolic subgroup P of a semisimple Lie group as a product P=MAN of a reductive subgroup M, an abelian subgroup A, and a nilpotent subgroup N.- Applications :...
of a parabolic subgroup.

