In the theory of dynamical system

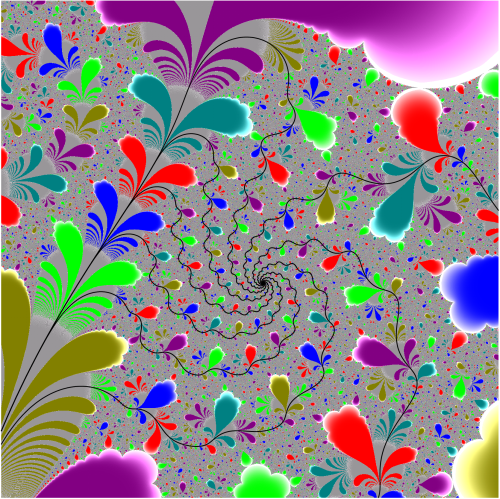
Dynamical system

A dynamical system is a concept in mathematics where a fixed rule describes the time dependence of a point in a geometrical space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, and the number of fish each springtime in a...

s, the exponential map

Exponential function

In mathematics, the exponential function is the function ex, where e is the number such that the function ex is its own derivative. The exponential function is used to model a relationship in which a constant change in the independent variable gives the same proportional change In mathematics,...

 can be used as the evolution function

Dynamical system (definition)

The dynamical system concept is a mathematical formalization for any fixed "rule" which describes the time dependence of a point's position in its ambient space...

 of the discrete nonlinear dynamical system

Dynamical system

A dynamical system is a concept in mathematics where a fixed rule describes the time dependence of a point in a geometrical space. Examples include the mathematical models that describe the swinging of a clock pendulum, the flow of water in a pipe, and the number of fish each springtime in a...

.

The family of exponential functions is called exponential family.

There are many forms of these maps , many of which are equivalent under a coordinate transformation. For example two of the most common ones are:

• 
• 

The second one can be mapped to the first using the fact that 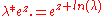, so 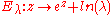is the same under the transformation . The only difference is that, due to multi-valued properties of exponentiation, there may be a few select cases that can only be found in one version. Similar arguments can be made for many other formulas.