Daniel Goldston
Encyclopedia
Daniel Alan Goldston is an American
mathematician
who specializes in number theory
. He is currently a professor of mathematics at San Jose State University
.
Goldston is best known for the following result that he, János Pintz
, and Cem Yıldırım
proved in 2005:
where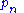
denotes the nth prime number
. In other words, for every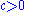
, there exist infinitely many pairs of consecutive primes 
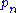and 
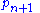which are closer to each other than the average distance between consecutive primes by a factor of 
, i.e.,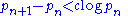 
.
This result was originally reported in 2003 by Dan Goldston and Cem Yıldırım but was later retracted. Then Janos Pintz joined the team and they completed the proof in 2005.
In fact, if they assume the Elliott-Halberstam conjecture
, then they can also show that primes within 16 of each other occur infinitely often, which is related to the twin prime conjecture
.
United States
The United States of America is a federal constitutional republic comprising fifty states and a federal district...
mathematician
Mathematician
A mathematician is a person whose primary area of study is the field of mathematics. Mathematicians are concerned with quantity, structure, space, and change....
who specializes in number theory
Number theory
Number theory is a branch of pure mathematics devoted primarily to the study of the integers. Number theorists study prime numbers as well...
. He is currently a professor of mathematics at San Jose State University
San José State University
San Jose State University is a public university located in San Jose, California, United States...
.
Goldston is best known for the following result that he, János Pintz
János Pintz
János Pintz is a Hungarian mathematician working in analytic number theory. He is a fellow of the Rényi Mathematical Institute and is also a member of the Hungarian Academy of Sciences.-Mathematical results:...
, and Cem Yıldırım
Cem Yildirim
Cem Yalçın Yıldırım is a Turkish mathematician who specializes in number theory. He obtained his PhD from the University of Toronto in 1990. His advisor was John Friedlander...
proved in 2005:
where
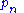denotes the nth prime numberPrime number
A prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. A natural number greater than 1 that is not a prime number is called a composite number. For example 5 is prime, as only 1 and 5 divide it, whereas 6 is composite, since it has the divisors 2...
. In other words, for every
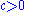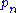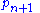, there exist infinitely many pairs of consecutive primes and which are closer to each other than the average distance between consecutive primes by a factor of , i.e., 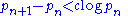.This result was originally reported in 2003 by Dan Goldston and Cem Yıldırım but was later retracted. Then Janos Pintz joined the team and they completed the proof in 2005.
In fact, if they assume the Elliott-Halberstam conjecture
Elliott-Halberstam conjecture
In number theory, the Elliott–Halberstam conjecture is a conjecture about the distribution of prime numbers in arithmetic progressions. It has many applications in sieve theory. It is named for Peter D. T. A. Elliott and Heini Halberstam....
, then they can also show that primes within 16 of each other occur infinitely often, which is related to the twin prime conjecture
Twin prime
A twin prime is a prime number that differs from another prime number by two. Except for the pair , this is the smallest possible difference between two primes. Some examples of twin prime pairs are , , , , and...
.