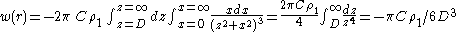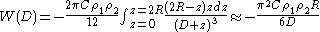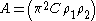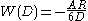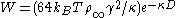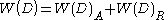DLVO theory
Encyclopedia
The DLVO theory is named after Derjaguin
and Landau, Verwey and Overbeek.
The theory describes the force between charged surfaces interacting through a liquid medium.
It combines the effects of the van der Waals
attraction and the electrostatic repulsion due to the so called double layer
of counterion
s.
The electrostatic part of the DLVO interaction is computed in the mean field approximation in the limit of low surface potentials - that is when the potential energy of an elementary charge on the surface is much smaller than the thermal energy scale,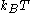 .
.
For two spheres of radius with constant surface charge
with constant surface charge 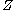
separated by a center-to-center distance in a fluid of
in a fluid of
dielectric constant
 containing a concentration
containing a concentration  of monovalent ions,
of monovalent ions,
the electrostatic potential takes the form of a screened-Coulomb or Yukawa repulsion
,
Boris Derjaguin
Professor Boris Vladimirovich Derjaguin was one of the renowned Soviet/Russian chemists of the twentieth century. As a member of the Russian Academy of Sciences he laid the foundation of the modern science of colloids and surfaces...
and Landau, Verwey and Overbeek.
The theory describes the force between charged surfaces interacting through a liquid medium.
It combines the effects of the van der Waals
Van der Waals force
In physical chemistry, the van der Waals force , named after Dutch scientist Johannes Diderik van der Waals, is the sum of the attractive or repulsive forces between molecules other than those due to covalent bonds or to the electrostatic interaction of ions with one another or with neutral...
attraction and the electrostatic repulsion due to the so called double layer
Double layer (interfacial)
A double layer is a structure that appears on the surface of an object when it is placed into a liquid. The object might be a solid particle, a gas bubble, a liquid droplet, or a porous body. The DL refers to two parallel layers of charge surrounding the object...
of counterion
Counterion
A counterion is the ion that accompanies an ionic species in order to maintain electric neutrality. In table salt the sodium cation is the counterion for the chlorine anion and vice versa.In a charged transition metal complex, a simple A counterion is the ion that accompanies an ionic species in...
s.
The electrostatic part of the DLVO interaction is computed in the mean field approximation in the limit of low surface potentials - that is when the potential energy of an elementary charge on the surface is much smaller than the thermal energy scale,
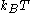 .
.For two spheres of radius
 with constant surface charge
with constant surface charge 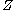
separated by a center-to-center distance
 in a fluid of
in a fluid ofdielectric constant
Dielectric constant
The relative permittivity of a material under given conditions reflects the extent to which it concentrates electrostatic lines of flux. In technical terms, it is the ratio of the amount of electrical energy stored in a material by an applied voltage, relative to that stored in a vacuum...
 containing a concentration
containing a concentration  of monovalent ions,
of monovalent ions,the electrostatic potential takes the form of a screened-Coulomb or Yukawa repulsion
Yukawa interaction
In particle physics, Yukawa's interaction, named after Hideki Yukawa, is an interaction between a scalar field \phi and a Dirac field \Psi of the typeV \approx g\bar\Psi \phi \Psi or g \bar \Psi \gamma^5 \phi \Psi ....
,
-
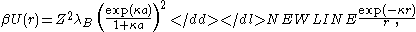
where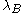 is the Bjerrum lengthBjerrum lengthThe Bjerrum length is the separation at which the electrostatic interaction between twoelementary charges is comparable in magnitude to the thermal energy scale,...
is the Bjerrum lengthBjerrum lengthThe Bjerrum length is the separation at which the electrostatic interaction between twoelementary charges is comparable in magnitude to the thermal energy scale,...
,
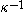 is the Debye-Hückel screening length,
is the Debye-Hückel screening length,
which is given by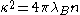 , and
, and
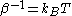 is the thermal energy scale at absolute temperature
is the thermal energy scale at absolute temperature 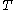 .
.
History
In 1923, Debye and HückelDebye-Hückel equationThe Debye–Hückel equation and Debye–Hückel limiting law, were derived by Peter Debye and Erich Hückel, who developed a theory with which to calculate activity coefficients of electrolyte solutions. Activities, rather than concentrations, are needed in many chemical calculations because solutions...
reported the first successful
theory for the distribution of charges in ionic solutions.
The framework of linearized Debye-Hückel theory subsequently
was applied to colloidal dispersions by Levine and Dube
who found that charged colloidal particles should
experience a strong medium-range repulsion and a weaker long-range attraction.
This theory did not explain the observed instability of colloidal dispersions against
irreversible aggregation in solutions of high ionic strength.
In 1941, DerjaguinBoris DerjaguinProfessor Boris Vladimirovich Derjaguin was one of the renowned Soviet/Russian chemists of the twentieth century. As a member of the Russian Academy of Sciences he laid the foundation of the modern science of colloids and surfaces...
and Landau
introduced a theory for the stability of colloidal dispersions that invoked
a fundamental instability driven by strong but short-ranged
van der Waals attractions countered by the
stabilizing influence of electrostatic repulsions.
Seven years later, Verwey and
Overbeek independently arrived at the same result.
This so-called DLVO theory resolved the failure of the
Levine-Dube theory to account
for the dependence of colloidal dispersions' stability
on the ionic strength of the electrolyte.
Derivation of DLVO theory
DLVO theory is the combined effect of van der WaalsVan der Waals forceIn physical chemistry, the van der Waals force , named after Dutch scientist Johannes Diderik van der Waals, is the sum of the attractive or repulsive forces between molecules other than those due to covalent bonds or to the electrostatic interaction of ions with one another or with neutral...
and double layerDouble layer (interfacial)A double layer is a structure that appears on the surface of an object when it is placed into a liquid. The object might be a solid particle, a gas bubble, a liquid droplet, or a porous body. The DL refers to two parallel layers of charge surrounding the object...
force. For the derivation, different conditions must be taken into account and different equations can be obtained. But some useful assumptions can effectively simplify the process, which are suitable for ordinary conditions. The simplified way to derive it is to add the two parts together.
van der Waals attraction
van der Waals force is actually the total name of dipole-dipole force, dipole-induced dipole force and dispersion forces, in which dispersion forces are the most important part because they are always present.
Assume that the pair potential between two atoms or small molecules is purely attractive and of the form w = -C/rn, where C is a constant for interaction energy, decided by the molecule's property and n = 6 for van der Waals attraction. With another assumption of additivity, the net interaction energy between a molecule and planar surface made up of like molecules will be the sum of the interaction energy between the molecule and every molecule in the surface body. So the net interaction energy for a molecule at a distance D away from the surface will therefore be
where-
- w(r) is the interaction energy between the molecule and the surface
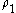 is the number density of the surface.
is the number density of the surface.- z is the axis perpendicular with the surface and passes across the molecule. z = 0 at the point where the molecule is and z = D at the surface.
- x is the axis perpendicular with z axis, where x = 0 at the intersection.
Then the interaction energy of a large sphere of radius R and a flat surface can be calculated as
where-
- W(D) is the interaction energy between the sphere and the surface.
-
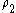 is the number density of the sphere
is the number density of the sphere
For convenience, Hamaker constantHamaker ConstantThe Hamaker constant A can be defined for a Van der Waals body-body interaction:A=\pi^2\times C \times \rho_1 \times \rho_2where \rho_1 and \rho_2 are the number of atoms per unit volume in two interacting bodies and C is the coefficient in the particle-particle pair interaction.The Hamaker...
A is given as
and the equation will become
With a similar method and according to Derjaguin approximation, the van der Waals interaction energy between particles with different shapes can be calculated, such as energy between
- two spheres:
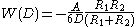
- sphere-surface:
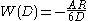
- Two surfaces:
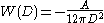 per unit area
per unit area
Double layer force
A surface in a liquid may be charged by dissociation of surface groups (e.g. silanol groups for glass or silica surfaces
) or by adsorption of charged molecules such as polyelectrolyte from the surrounding solution. This results in the development of a wall surface potential which will attract counterions from the surrounding solution and repel co-ions. In equilibrium, the surface charge is balanced by oppositely charged counterions in solution. The region near the surface of enhanced
counterion concentration is called the electrical double layer (EDL). The EDL can be approximated by a sub-division into two regions. Ions in the region closest to the charged wall surface are strongly bound to the surface. This immobile layer is called the Stern or Helmholtz layer. The region adjacent to the Stern layer is called the diffuse layer and contains loosely associated ions that are comparatively mobile. The total electrical double layer due to the formation of the counterion layers results in electrostatic screening of the wall charge and minimizes the Gibbs free energyGibbs free energyIn thermodynamics, the Gibbs free energy is a thermodynamic potential that measures the "useful" or process-initiating work obtainable from a thermodynamic system at a constant temperature and pressure...
of EDL formation.
The thickness of the diffuse electric double layer,is known as the Debye screening lengthDouble layer (interfacial)A double layer is a structure that appears on the surface of an object when it is placed into a liquid. The object might be a solid particle, a gas bubble, a liquid droplet, or a porous body. The DL refers to two parallel layers of charge surrounding the object...
 . At a distance of two Debye screening lengths the electrical potential energy is reduced to 2 percent of the value at the surface wall.
. At a distance of two Debye screening lengths the electrical potential energy is reduced to 2 percent of the value at the surface wall.
with unit of m−1
where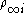 is the number density of ion i in the bulk solution.
is the number density of ion i in the bulk solution.- z is the valency of the ion. For example, H+ has a valency of +1 and Ca2+ has a valency of +2.
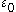 is the electric constantElectric constantThe physical constant ε0, commonly called the vacuum permittivity, permittivity of free space or electric constant is an ideal, physical constant, which is the value of the absolute dielectric permittivity of classical vacuum...
is the electric constantElectric constantThe physical constant ε0, commonly called the vacuum permittivity, permittivity of free space or electric constant is an ideal, physical constant, which is the value of the absolute dielectric permittivity of classical vacuum...
, is the relative static permittivity.
is the relative static permittivity.- kB is the Boltzmann constant.
The repulsive free energy per unit area between two planar surfaces is shown as
where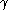 is the reduced surface potential
is the reduced surface potential

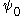 is the potential on the surface.
is the potential on the surface.
The interaction free energy between two spheres of radius R is
-
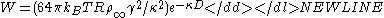
Combining the van der Waals interaction energy and the double layer interaction energy, the interaction between two particles or two surfaces in a liquid can be expressed as:
where W(D)R is the repulsive interaction energy due two electric repulsion and W(D)A is the attractive interaction energy due to van der Waals interaction.
Application of DLVO theory
Since 1940s, the DLVO theory has been used to explain phenomena found in colloidal science, adsorption and many other fields. Due to the appearance of nanoparticles, DLVO theory becomes even more popular. Because it can be used to explain both general nanoparticles such as fullerenes particles and microorganismsBacterial adhesion in aquatic systemBacterial adhesion involves the attachment of bacteria on the surface . This interaction plays an important role in natural system as well as in environmental engineering....
.
Shortcomings of the DLVO theory
The theory is not effective in describing ordering processes such as the evolution of colloidal crystalColloidal crystalA colloidal crystal is an ordered array of colloid particles, analogous to a standard crystal whose repeating subunits are atoms or molecules. A natural example of this phenomenon can be found in the gem opal, where spheres of silica assume a close-packed locally periodic structure under moderate...
s in dilute dispersions with low salt concentrations. It also can not explain the relation between the formation of colloidal crystals and salt concentrations. -
-