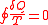
Clausius theorem
Encyclopedia
Overview
The ClausiusRudolf Clausius
Rudolf Julius Emanuel Clausius , was a German physicist and mathematician and is considered one of the central founders of the science of thermodynamics. By his restatement of Sadi Carnot's principle known as the Carnot cycle, he put the theory of heat on a truer and sounder basis...
theorem (1855) states that in a cyclic process
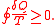
The equality holds in the reversible case and the '<' is in the irreversible case. The reversible case is used to introduce the state function entropy
Entropy
Entropy is a thermodynamic property that can be used to determine the energy available for useful work in a thermodynamic process, such as in energy conversion devices, engines, or machines. Such devices can only be driven by convertible energy, and have a theoretical maximum efficiency when...
. This is because in cyclic process the variation of a state function is zero.
The Clausius Theorem is a mathematical explanation of the Second Law of Thermodynamics. Also referred to as the “Inequality of Clausius”, the theorem was developed by Rudolph Clausius and intends to explain the relationship between the heat flow in a system and the entropy of the system and its surroundings. Clausius developed this in his efforts to explain entropy and define it quantitatively. In more direct terms, the theorem gives us a way to determine if a cyclical process is reversible or irreversible. The Clausius Theorem provides a quantitative formula for understanding the second law.
Clausius was one of the first to work on the idea of entropy and is even responsible for giving it that name. What is now known as the Clausius Theorem was first published in 1862 in Clausius’ sixth memoir, “On the Application of the Theorem of the Equivalence of Transformations to Interior Work”. Clausius sought to show a proportional relationship between entropy and the heat flow (dQ) through a system. In a system, heat can be transformed into work, and work can be transformed into heat through a cyclical process. Clausius writes that “The algebraic sum of all the transformations occurring in a cyclical process can only be positive, or, as an extreme case, equal to nothing.” In other words, the equation
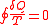
(where dQ is heat flow given up by the body to a reservoir and T is absolute temperature of the body when this heat is given up) must be found to be true for any process that is cyclical and reversible. He then took this a step further and determined that the following equation must be found true for any cyclical process that is possible, reversible or not. This equation is the “Clausius Inequality”.
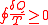
Now that this is known, there must be a relation developed between the Clausius Inequality and entropy. The change in entropy S is defined as
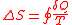
Using all of equations above, we see that the change in entropy gives us insight into whether or not a cyclical system in reversible or irreversible. In any thermodynamic process, you can have both positive and negative transformations of heat and work. For a reversible process, the positive and negative transformations must balance out and equal zero. For irreversible processes, the positive and negative transformations do not necessarily have to equal zero, but the positive changes must outweigh the negative changes. If the change in entropy is greater than zero, then the process is irreversible. If the change in entropy is equal to zero, then the process is reversible. A negative change in entropy is not possible. This directly ties into the Second Law of Thermodynamics, which states that “The entropy of the Universe is increasing”. It must be emphasized that the entropy of a system is a state function. This means that it depends only on what state the system is currently in, and not what path the system took to get there.
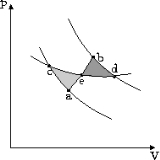
Proof
Suppose a system absorbs heat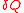 at temperature
at temperature  . Since the value of
. Since the value of 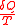 does not depend on the details of how the heat is transferred, we can assume it is from a Carnot engine, which in turn absorbs heat
does not depend on the details of how the heat is transferred, we can assume it is from a Carnot engine, which in turn absorbs heat 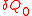 from a heat reservoir with constant temperature
from a heat reservoir with constant temperature  .
.According to the nature of Carnot cycle
Carnot cycle
The Carnot cycle is a theoretical thermodynamic cycle proposed by Nicolas Léonard Sadi Carnot in 1824 and expanded by Benoit Paul Émile Clapeyron in the 1830s and 40s. It can be shown that it is the most efficient cycle for converting a given amount of thermal energy into work, or conversely,...
,
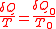
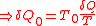
Therefore in one cycle, the total heat absorbed from the reservoir is

Since after a cycle, the system and the Carnot engine as a whole return to its initial status, the difference of the internal energy is zero. Thus according to First Law of Thermodynamics
First law of thermodynamics
The first law of thermodynamics is an expression of the principle of conservation of work.The law states that energy can be transformed, i.e. changed from one form to another, but cannot be created nor destroyed...
,

According to the Kelvin statement of Second Law of thermodynamics
Second law of thermodynamics
The second law of thermodynamics is an expression of the tendency that over time, differences in temperature, pressure, and chemical potential equilibrate in an isolated physical system. From the state of thermodynamic equilibrium, the law deduced the principle of the increase of entropy and...
, we cannot drain heat from one reservoir and convert them entirely into work without making any other changes, so

Combine all the above and we get Clausius inequality
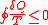
If the system is reversible
Reversible process (thermodynamics)
In thermodynamics, a reversible process, or reversible cycle if the process is cyclic, is a process that can be "reversed" by means of infinitesimal changes in some property of the system without loss or dissipation of energy. Due to these infinitesimal changes, the system is in thermodynamic...
, then reverse its path and do the experiment again we can get

Thus