Whipple formulae
Encyclopedia
In the theory of special functions
, Whipple's transformation for Legendre functions, named after Francis John Welsh Whipple
, arise from a general expression, concerning associated Legendre functions. These formulae have been presented previously in terms of a viewpoint aimed at spherical harmonic
s, now that we view the equations in terms of toroidal coordinates
, whole new symmetries of Legendre functions arise.
For associated Legendre functions of the first and second kind,
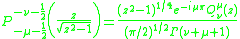
and
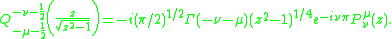
These expressions are valid for all parameters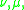
and 
. By shifting the complex degree and order in an appropriate fashion, we obtain Whipple formulae for general complex index interchange of general associated Legendre functions of the first and second kind. These are given by
and
Note that these formulae are well-behaved for all values of the degree and order, except for those with integer values. However, if we examine these formulae for toroidal harmonics, i.e. where the degree is half-integer, the order is integer, and the argument is positive and greater than unity one obtains
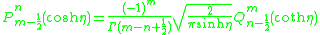
and
.
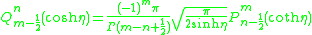These are the Whipple formulae for toroidal harmonics. They show an important property of toroidal harmonics under index (the integers associated with the order and the degree) interchange.
Special functions
Special functions are particular mathematical functions which have more or less established names and notations due to their importance in mathematical analysis, functional analysis, physics, or other applications....
, Whipple's transformation for Legendre functions, named after Francis John Welsh Whipple
Francis John Welsh Whipple
Francis John Welsh Whipple was a British mathematician and meteorologist. From 1925 to 1939 he was superintendent of the Kew Observatory.-Biography:...
, arise from a general expression, concerning associated Legendre functions. These formulae have been presented previously in terms of a viewpoint aimed at spherical harmonic
Spherical Harmonic
Spherical Harmonic is a science fiction novel from the Saga of the Skolian Empire by Catherine Asaro. It tells the story of Dyhianna Selei , the Ruby Pharaoh of the Skolian Imperialate, as she strives to reform her government and reunite her family in the aftermath of a devastating interstellar...
s, now that we view the equations in terms of toroidal coordinates
Toroidal coordinates
Toroidal coordinates are a three-dimensional orthogonal coordinate system that results from rotating the two-dimensional bipolar coordinate system about the axis that separates its two foci. Thus, the two foci F_1 and F_2 in bipolar coordinates become a ring of radius a in the xy plane of the...
, whole new symmetries of Legendre functions arise.
For associated Legendre functions of the first and second kind,
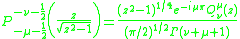
and
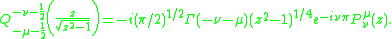
These expressions are valid for all parameters
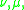and . By shifting the complex degree and order in an appropriate fashion, we obtain Whipple formulae for general complex index interchange of general associated Legendre functions of the first and second kind. These are given by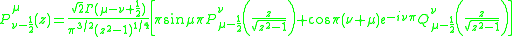and
Note that these formulae are well-behaved for all values of the degree and order, except for those with integer values. However, if we examine these formulae for toroidal harmonics, i.e. where the degree is half-integer, the order is integer, and the argument is positive and greater than unity one obtains
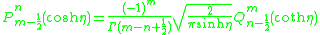
and
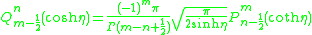.These are the Whipple formulae for toroidal harmonics. They show an important property of toroidal harmonics under index (the integers associated with the order and the degree) interchange.