
Thompson order formula
Encyclopedia
In mathematical finite group theory, the Thompson order formula, introduced by John Griggs Thompson, gives a formula for the order of a finite group in terms of the centralizers of involutions.
Here a(x) is the number of pairs (u,v) with u conjugate to t, v conjugate to z, and x in the subgroup generated by uv.
There are also more complicated versions of the Thompson order formula for the case when G has more than two conjugacy classes of involution.
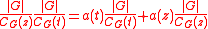
The left hand side just counts the number of pairs on involutions (u,v) with u conjugate to t, v conjugate to z. The right hand side counts these in two classes, depending on whether the involution in the cyclic group generated by uv is conjugate to t or z.
Statement
If a finite group G has exactly two conjugacy classes of involutions with representatives t and z, then the Thompson order formula states- |G| = |CG(z)|a(t) + |CG(t)|a(z)
Here a(x) is the number of pairs (u,v) with u conjugate to t, v conjugate to z, and x in the subgroup generated by uv.
There are also more complicated versions of the Thompson order formula for the case when G has more than two conjugacy classes of involution.
Proof
The Thompson order formula can be rewritten as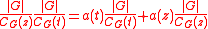
The left hand side just counts the number of pairs on involutions (u,v) with u conjugate to t, v conjugate to z. The right hand side counts these in two classes, depending on whether the involution in the cyclic group generated by uv is conjugate to t or z.

