Structure implies multiplicity
Encyclopedia
In diatonic set theory
structure implies multiplicity is a quality of a collection or scale. This is that for the interval series formed by the shortest distance around a diatonic circle of fifths
between member of a series indicates the number of unique interval
patterns (adjacently, rather than around the circle of fifths) formed by diatonic transpositions of that series. Structure being the intervals in relation to the circle of fifths, multiplicity being the number of times each different (adjacent) interval pattern occurs. The property was first described by John Clough
and Gerald Myerson in "Variety and Multiplicity in Diatonic Systems" (1985). (Johnson 2003, p.68, 151)
Structure implies multiplicity is true of the diatonic collection and the pentatonic scale
, and any subset.
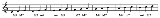
For example, cardinality equals variety
dictates that a three member diatonic subset of the C major scale, C-D-E transposed to all scale degrees gives three interval patterns: M2-M2, M2-m2, m2-M2.
 On the circle of fifths:
On the circle of fifths:
C G D A E B F (C)
1 2 1 2 1 2 3
E and C are three notes apart, C and D are two notes apart, D and E two notes apart. Just as the distance around the circle of fifths between forms the interval pattern 3-2-2, M2-M2 occurs three times, M2-m2 occurs twice, and m2-M2 occurs twice.
Cardinality equals variety
and structure implies multiplicity are true of all collections with Myhill's property
or maximal evenness
.
Diatonic set theory
Diatonic set theory is a subdivision or application of musical set theory which applies the techniques and insights of discrete mathematics to properties of the diatonic collection such as maximal evenness, Myhill's property, well formedness, the deep scale property, cardinality equals variety, and...
structure implies multiplicity is a quality of a collection or scale. This is that for the interval series formed by the shortest distance around a diatonic circle of fifths
Circle of fifths
In music theory, the circle of fifths shows the relationships among the 12 tones of the chromatic scale, their corresponding key signatures, and the associated major and minor keys...
between member of a series indicates the number of unique interval
Interval (music)
In music theory, an interval is a combination of two notes, or the ratio between their frequencies. Two-note combinations are also called dyads...
patterns (adjacently, rather than around the circle of fifths) formed by diatonic transpositions of that series. Structure being the intervals in relation to the circle of fifths, multiplicity being the number of times each different (adjacent) interval pattern occurs. The property was first described by John Clough
John Clough
John Clough born September 13, 1984 is a rugby league player who currently plays for the Blackpool Panthers rugby league team. Clough is the club Captain. A former Lancashire Academy representative, John plays at hooker. John was born in St Helens and is brother of St Helens player Paul...
and Gerald Myerson in "Variety and Multiplicity in Diatonic Systems" (1985). (Johnson 2003, p.68, 151)
Structure implies multiplicity is true of the diatonic collection and the pentatonic scale
Pentatonic scale
A pentatonic scale is a musical scale with five notes per octave in contrast to a heptatonic scale such as the major scale and minor scale...
, and any subset.
For example, cardinality equals variety
Cardinality equals variety
The musical operation of scalar transposition shifts every note in a melody by the same number of scale steps. The musical operation of chromatic transposition shifts every note in a melody by the same distance in pitch class space...
dictates that a three member diatonic subset of the C major scale, C-D-E transposed to all scale degrees gives three interval patterns: M2-M2, M2-m2, m2-M2.

C G D A E B F (C)
1 2 1 2 1 2 3
E and C are three notes apart, C and D are two notes apart, D and E two notes apart. Just as the distance around the circle of fifths between forms the interval pattern 3-2-2, M2-M2 occurs three times, M2-m2 occurs twice, and m2-M2 occurs twice.
Cardinality equals variety
Cardinality equals variety
The musical operation of scalar transposition shifts every note in a melody by the same number of scale steps. The musical operation of chromatic transposition shifts every note in a melody by the same distance in pitch class space...
and structure implies multiplicity are true of all collections with Myhill's property
Myhill's property
In diatonic set theory Myhill's property is the quality of musical scales or collections with exactly two specific intervals for every generic interval, and thus also have the properties of maximal evenness, cardinality equals variety, structure implies multiplicity, and be a well formed generated...
or maximal evenness
Maximal evenness
In diatonic set theory maximal evenness is the quality of a collection or scale which for every generic interval there are either one or two consecutive specific intervals, in other words a scale which is "spread out as much as possible." This property was first described by music theorist John...
.
Further reading
- Clough, John and Myerson, Gerald (1985). "Variety and Multiplicity in Diatonic Systems", Journal of Music Theory 29: 249-70.
- Agmon, Eytan (1989). "A Mathematical Model of the Diatonic System", Journal of Music Theory 33: 1-25.
- Agmon, Eytan (1996). "Coherent Tone-Systems: A Study in the Theory of Diatonicism", Journal of Music Theory 40: 39-59.
Source
- Johnson, Timothy (2003). Foundations of Diatonic Theory: A Mathematically Based Approach to Music Fundamentals. Key College Publishing. ISBN 1-930190-80-8.

