
Slater's rules
Encyclopedia
In quantum chemistry
, Slater's rules provide numerical values for the effective nuclear charge
concept. In a many-electron atom, each electron is said to experience less than the actual nuclear charge
owning to shielding
or screening
by the other electrons. For each electron in an atom, Slater's rules provide a value for the screening constant, denoted by s, S, or σ, which relates the effective and actual nuclear charges as
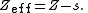
The rules were devised semi-empirically by John C. Slater
and published in 1930.
Updated values of screening constants were provided by Clementi et al.
n, and for equal n in order of increasing azimuthal quantum number
l, except that s- and p- orbitals are kept together.
Each group is given a different shielding constant which depends upon the number and types of electrons in those groups preceding it.
The shielding constant for each group is formed as the sum of the following contributions:
In tabular form, the rules are summarized as:
atom which has nuclear charge 26 and electronic configuration 1s22s22p63s23p63d64s2. The screening constant, and subsequently the effective nuclear charge for each electron is deduced as:
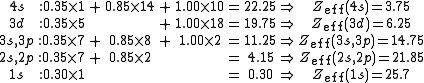
of any electron in an atom. Specifically, for each electron in an atom, Slater wished to determine shielding constants (s) and "effective" quantum numbers (n*) such that
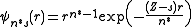
provides a reasonable approximation to a single-electron wave function. Slater defined n* by the rule that for n = 1, 2, 3, 4, 5, 6 respectively; n* = 1, 2, 3, 3.7, 4.0 and 4.2. This was an arbitrary adjustment to fit calculated atomic energies to experimental data.
Such a form was inspired by the known wave function spectrum of hydrogen-like atom
s which have the radial component
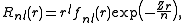
where n is the (true) principal quantum number
, l the azimuthal quantum number
, and fnl(r) is an oscillatory polynomial with n - l - 1 nodes. Slater argued on the basis of previous calculations by Clarence Zener
that the presence of radial nodes was not required to obtain a reasonable approximation. He also noted that in the asymptotic limit (far away from the nucleus), his approximate form coincides with the exact hydrogen-like wave function in the presence of a nuclear charge of Z-s and in the state with a principal quantum number n equal to his effective quantum number n*.
Slater then argued, again based on the work of Zener, that the total energy of a N-electron atom with a wavefunction constructed from orbitals of his form should be well approximated as
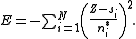
Using this expression for the total energy of an atom (or ion) as a function of the shielding constants and effective quantum numbers, Slater was able to compose rules such that spectral energies calculated agree reasonably well with experimental values for a wide range of atoms. Using the values in the iron example above, the total energy of a neutral iron atom using this method is -2497.2 Ry
, while the energy of an iron cation lacking a single 1s electron is -1964.6 Ry. The difference, 532.6 Ry, can be compared to the experimental (circa 1930) K absorption limit of 524.0 Ry.
Quantum chemistry
Quantum chemistry is a branch of chemistry whose primary focus is the application of quantum mechanics in physical models and experiments of chemical systems...
, Slater's rules provide numerical values for the effective nuclear charge
Effective nuclear charge
The effective nuclear charge is the net positive charge experienced by an electron in a multi-electron atom. The term "effective" is used because the shielding effect of negatively charged electrons prevents higher orbital electrons from experiencing the full nuclear charge by the repelling effect...
concept. In a many-electron atom, each electron is said to experience less than the actual nuclear charge
Atomic nucleus
The nucleus is the very dense region consisting of protons and neutrons at the center of an atom. It was discovered in 1911, as a result of Ernest Rutherford's interpretation of the famous 1909 Rutherford experiment performed by Hans Geiger and Ernest Marsden, under the direction of Rutherford. The...
owning to shielding
Shielding effect
The shielding effect describes the decrease in attraction between an electron and the nucleus in any atom with more than one electron shell. It is also referred to as the screening effect or atomic shielding.-Cause:...
or screening
Electric field screening
Screening is the damping of electric fields caused by the presence of mobile charge carriers. It is an important part of the behavior of charge-carrying fluids, such as ionized gases and conduction electrons in semiconductors and metals....
by the other electrons. For each electron in an atom, Slater's rules provide a value for the screening constant, denoted by s, S, or σ, which relates the effective and actual nuclear charges as
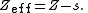
The rules were devised semi-empirically by John C. Slater
John C. Slater
John Clarke Slater was a noted American physicist who made major contributions to the theory of the electronic structure of atoms, molecules and solids. This work is of ongoing importance in chemistry, as well as in many areas of physics. He also made major contributions to microwave electronics....
and published in 1930.
Updated values of screening constants were provided by Clementi et al.
Rules
Firstly, the electrons are arranged in to a sequence of groups in order of increasing principal quantum numberPrincipal quantum number
In atomic physics, the principal quantum symbolized as n is the firstof a set of quantum numbers of an atomic orbital. The principal quantum number can only have positive integer values...
n, and for equal n in order of increasing azimuthal quantum number
Azimuthal quantum number
The azimuthal quantum number is a quantum number for an atomic orbital that determines its orbital angular momentum and describes the shape of the orbital...
l, except that s- and p- orbitals are kept together.
- [1s] [2s,2p] [3s,3p] [3d] [4s,4p] [4d] [4f] [5s, 5p] [5d] etc.
Each group is given a different shielding constant which depends upon the number and types of electrons in those groups preceding it.
The shielding constant for each group is formed as the sum of the following contributions:
- An amount of 0.35 from each other electron within the same group except for the [1s] group, where the other electron contributes only 0.30.
- If the group is of the [s p] type, an amount of 0.85 from each electron with principal quantum number (n) one less and an amount of 1.00 for each electron with an even smaller principal quantum number
- If the group is of the [d] or [f], type, an amount of 1.00 for each electron inside it. This includes i) electrons with a smaller principal quantum number and ii) electrons with an equal principal quantum number and a smaller azimuthal quantum numberAzimuthal quantum numberThe azimuthal quantum number is a quantum number for an atomic orbital that determines its orbital angular momentum and describes the shape of the orbital...
(l)
In tabular form, the rules are summarized as:
| Group | Other electrons in the same group | Electrons in group(s) with principal quantum number Principal quantum number In atomic physics, the principal quantum symbolized as n is the firstof a set of quantum numbers of an atomic orbital. The principal quantum number can only have positive integer values... n and azimuthal quantum number Azimuthal quantum number The azimuthal quantum number is a quantum number for an atomic orbital that determines its orbital angular momentum and describes the shape of the orbital... < l |
Electrons in group(s) with principal quantum number Principal quantum number In atomic physics, the principal quantum symbolized as n is the firstof a set of quantum numbers of an atomic orbital. The principal quantum number can only have positive integer values... n-1 |
Electrons in all group(s) with principal quantum number Principal quantum number In atomic physics, the principal quantum symbolized as n is the firstof a set of quantum numbers of an atomic orbital. The principal quantum number can only have positive integer values... < n-1 |
|---|---|---|---|---|
| [1s] | 0.3 | N/A | N/A | N/A |
| [ns,np] | 0.35 | N/A | 0.85 | 1 |
| [nd] or [nf] | 0.35 | 1 | 1 | 1 |
Example
An example provided in Slater's original paper is for the ironIron
Iron is a chemical element with the symbol Fe and atomic number 26. It is a metal in the first transition series. It is the most common element forming the planet Earth as a whole, forming much of Earth's outer and inner core. It is the fourth most common element in the Earth's crust...
atom which has nuclear charge 26 and electronic configuration 1s22s22p63s23p63d64s2. The screening constant, and subsequently the effective nuclear charge for each electron is deduced as:
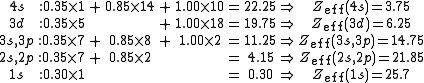
Motivation
The rules were developed by John C. Slater in an attempt to construct simple analytic expressions for the atomic orbitalAtomic orbital
An atomic orbital is a mathematical function that describes the wave-like behavior of either one electron or a pair of electrons in an atom. This function can be used to calculate the probability of finding any electron of an atom in any specific region around the atom's nucleus...
of any electron in an atom. Specifically, for each electron in an atom, Slater wished to determine shielding constants (s) and "effective" quantum numbers (n*) such that
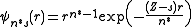
provides a reasonable approximation to a single-electron wave function. Slater defined n* by the rule that for n = 1, 2, 3, 4, 5, 6 respectively; n* = 1, 2, 3, 3.7, 4.0 and 4.2. This was an arbitrary adjustment to fit calculated atomic energies to experimental data.
Such a form was inspired by the known wave function spectrum of hydrogen-like atom
Hydrogen-like atom
A hydrogen-like ion is any atomic nucleus with one electron and thus is isoelectronic with hydrogen. Except for the hydrogen atom itself , these ions carry the positive charge e, where Z is the atomic number of the atom. Examples of hydrogen-like ions are He+, Li2+, Be3+ and B4+...
s which have the radial component
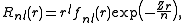
where n is the (true) principal quantum number
Principal quantum number
In atomic physics, the principal quantum symbolized as n is the firstof a set of quantum numbers of an atomic orbital. The principal quantum number can only have positive integer values...
, l the azimuthal quantum number
Azimuthal quantum number
The azimuthal quantum number is a quantum number for an atomic orbital that determines its orbital angular momentum and describes the shape of the orbital...
, and fnl(r) is an oscillatory polynomial with n - l - 1 nodes. Slater argued on the basis of previous calculations by Clarence Zener
Clarence Zener
Clarence Melvin Zener was the American physicist who first described the property concerning the breakdown of electrical insulators. These findings were later exploited by Bell Labs in the development of the Zener diode, which was duly named after him...
that the presence of radial nodes was not required to obtain a reasonable approximation. He also noted that in the asymptotic limit (far away from the nucleus), his approximate form coincides with the exact hydrogen-like wave function in the presence of a nuclear charge of Z-s and in the state with a principal quantum number n equal to his effective quantum number n*.
Slater then argued, again based on the work of Zener, that the total energy of a N-electron atom with a wavefunction constructed from orbitals of his form should be well approximated as
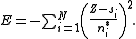
Using this expression for the total energy of an atom (or ion) as a function of the shielding constants and effective quantum numbers, Slater was able to compose rules such that spectral energies calculated agree reasonably well with experimental values for a wide range of atoms. Using the values in the iron example above, the total energy of a neutral iron atom using this method is -2497.2 Ry
Rydberg constant
The Rydberg constant, symbol R∞, named after the Swedish physicist Johannes Rydberg, is a physical constant relating to atomic spectra in the science of spectroscopy. Rydberg initially determined its value empirically from spectroscopy, but Niels Bohr later showed that its value could be calculated...
, while the energy of an iron cation lacking a single 1s electron is -1964.6 Ry. The difference, 532.6 Ry, can be compared to the experimental (circa 1930) K absorption limit of 524.0 Ry.

