In Schenkerian

Schenkerian analysis

Schenkerian analysis is a method of musical analysis of tonal music based on the theories of Heinrich Schenker. The goal of a Schenkerian analysis is to interpret the underlying structure of a tonal work. The theory's basic tenets can be viewed as a way of defining tonality in music...

 theory, a scale-step is a triad

Triad (music)

In music and music theory, a triad is a three-note chord that can be stacked in thirds. Its members, when actually stacked in thirds, from lowest pitched tone to highest, are called:* the Root...

 (based on one of the diatonic scale degrees) that is perceived as an organizing force for a passage of music (in accordance with the principle of composing-out). In Harmony

Harmony (Schenker)

Harmony is a book published in 1906 by Heinrich Schenker. It is the first installment of Schenker's three-volume treatise on music theory entitled New Musical Theories and Fantasies; the others are Counterpoint and Free Composition...

, Schenker

Schenker

Schenker is a German family name.Schenker may refer to:* Alexander M. Schenker , American slavist* Heinrich Schenker , Austrian music theorist* Rudolf Schenker, German guitarist, founding member of Scorpions...

 gives the following example and asserts that "our ear will connect the first tone, G, with the B on the first quarter of measure 1 as the third of G.

Likewise, it will connect that G with the D on the first quarter of measure 2 as its fifth. Our ear will establish this connection instinctively, but nonetheless in accordance with the demands of Nature. In an analogous way, it will link that first G with the C and E of the second half of measure 1 and thus form the concept of another triad. For our ear will miss no opportunity to hear such triads, no matter how far in the background of our consciousness this conception may lie hidden and no matter whether in the plan of the composition it is overshadowed by far more obvious and important relationships."

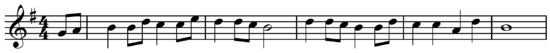
A scale-step triad is designated by an uppercase Roman numeral representing the scale degree of the root

Root (chord)

In music theory, the root of a chord is the note or pitch upon which a triadic chord is built. For example, the root of the major triad C-E-G is C....

, much as in traditional "harmonic analysis" (see chord progression

Chord progression

A chord progression is a series of musical chords, or chord changes that "aims for a definite goal" of establishing a tonality founded on a key, root or tonic chord. In other words, the succession of root relationships...

). Thus, in the above example (which is in G major

G major

G major is a major scale based on G, with the pitches G, A, B, C, D, E, and F. Its key signature has one sharp, F; in treble-clef key signatures, the sharp-symbol for F is usually placed on the first line from the top, though in some Baroque music it is placed on the first space from the bottom...

), the G major triad that Schenker claims we perceive through the first two measures would be labelled "I". However, unlike traditional harmonic analyses, Schenker's theory is not concerned with the mere labelling of such chords, but rather with discerning hierarchical relationships among tones. For Schenker, the chords occurring in a passage need not be of equal import. As he explains:
Furthermore, in terms of Schenker's mature theory, the question of whether a given triad possesses scale-step status depends on the structural level under discussion. Indeed, it follows from Schenker's concepts that, at the highest level, a tonal composition possesses only one scale step, since the entirety of the work may be understood as an elaboration of its tonic triad (i.e. scale-step I).