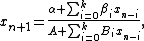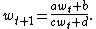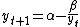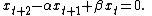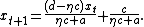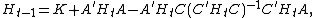Rational difference equation
Encyclopedia
A rational difference equation is a nonlinear difference equation of the form
where the initial conditions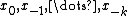
are such that the denominator is never zero for any 
.
When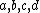
and the initial condition 
are real numbers, this difference equation is called a Riccati difference equation.
Such an equation can be solved by writing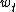
as a nonlinear transformation of another variable 
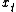which itself evolves linearly. Then standard methods can be used to solve the linear difference equation in 
.
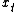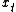, when 
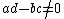, is to write
where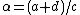
and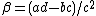 
and where 
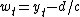. Further writing 
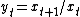can be shown to yield
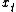instead of a second-order one, for the case in which 
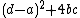is non-negative. Write 
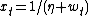implying 
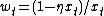, where 
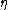is given by 
and where 
. Then it can be shown that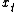 
evolves according to
which can arise in some discrete-time optimal control
problems, can be solved using the second approach above if the matrix C has only one more row than column.
where the initial conditions
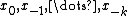are such that the denominator is never zero for any .First-order rational difference equation
A first-order rational difference equation is a nonlinear difference equation of the form
When
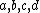and the initial condition are real numbers, this difference equation is called a Riccati difference equation.Such an equation can be solved by writing
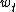as a nonlinear transformation of another variable 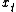which itself evolves linearly. Then standard methods can be used to solve the linear difference equation in 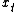.First approach
One approach to developing the transformed variable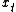 , when 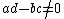, is to write
where
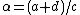and 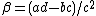and where 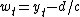. Further writing 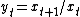can be shown to yield
Second approach
This approach gives a first-order difference equation for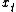 instead of a second-order one, for the case in which 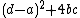is non-negative. Write 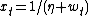implying 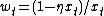, where 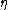is given by and where . Then it can be shown that 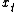evolves according to
Application
It was shown in that a dynamic matrix Riccati equation of the form
which can arise in some discrete-time optimal control
Optimal control
Optimal control theory, an extension of the calculus of variations, is a mathematical optimization method for deriving control policies. The method is largely due to the work of Lev Pontryagin and his collaborators in the Soviet Union and Richard Bellman in the United States.-General method:Optimal...
problems, can be solved using the second approach above if the matrix C has only one more row than column.
See also
- Newth, Gerald, "World order from chaotic beginnings," Mathematical Gazette 88, March 2004, 39-45, for a trigonometric approach.
- Simons, Stuart, "A non-linear difference equation," Mathematical Gazette 93, November 2009, 500-504.