
Quantum mechanical Bell test prediction
Encyclopedia
In physics
, the quantum mechanical Bell test prediction is the prediction that quantum mechanics would give for the correlation
probabilities for a set of measurements performed on a quantum entangled state. An important outcome of this prediction is that it violates the Bell inequality, which, as a result, has serious implications for the interpretation of quantum mechanics
.
The following is based on section 2 of the Stanford Encyclopedia of Philosophy
article written by Abner Shimony
, one of the authors of the original Clauser, Horne, Shimony and Holt article (1969) after which the CHSH Bell test is named (Shimony, 2004).
s A and B propagating respectively in the z and −z directions. The two kets |x>j and |y>j constitute a polarization
basis for photon j (j =A, B), the former representing (in Dirac's notation) a state in which the photon A is linearly polarized in the x-direction and the latter a state in which it is linearly polarized in the y-direction. For the two-photon system the four product kets |x>A |x>B, |x>A |y>B, |y>A |x>B, and |y>A |y>B constitute a polarization basis. Each two-photon polarization state can be expressed as a linear combination of these four basis states with complex coefficients. Of particular interest are the entangled quantum states, which in no way can be expressed as |φ>A|ξ>B, with |φ> and |ξ> single-photon states, an example being
which has the useful property of being invariant under rotation of the x and y axes in the plane perpendicular to z. Neither photon A nor photon B is in a definite polarization state when the pair is in the state |Φ>, but their potentialities (in the terminology of Heisenberg 1958) are correlated: if by measurement or some other process the potentiality of photon A to be polarized along the x-direction or along the y-direction is actualized, then the same will be true of photon B, and conversely.
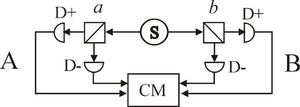 Suppose now that photons A and B impinge respectively on the faces of birefringent crystal
Suppose now that photons A and B impinge respectively on the faces of birefringent crystal
polarization analyzers, with the entrance face of each analyzer perpendicular to z. Each analyzer has the property of separating light incident upon its face into two outgoing non-parallel rays, the ordinary ray and the extraordinary ray. The transmission axis of the analyzer is a direction with the property that a photon polarized along it will emerge in the ordinary ray (with certainty if the crystals are assumed to be ideal), while a photon polarized in a direction perpendicular to z and to the transmission axis will emerge in the extraordinary ray. (See diagram.)
The crystals are also idealized by assuming that no incident photon is absorbed, but each emerges in either the '+' or the '−' channel. Quantum mechanics provides an algorithm for computing the probabilities that photons A and B will emerge from these idealized analyzers in specified rays, as functions of the orientations a and b of the analyzers, a being the angle between the transmission axis of the 'A' analyzer and an arbitrary fixed direction in the x–y plane, and b having the analogous meaning for B:
Here j is a quantum number
associated with the ray into which photon A emerges, taking values +1 or −1 depending on which channel it emerges from, while k is the analogous quantum number for photon B; and | <Φ|θj>A |ξk>B | is the ket representing the quantum state of photons A and B with the respective quantum numbers j and k. Calculation of the probabilities of interest from Eq. () can be simplified by using the invariance noted after Eq. () and rewriting |Φ> as
Eq. () results from Eq. () by substituting the transmission axis of the A analyzer for x and the direction perpendicular to both z and this transmission axis for y.
Since |θ−1>A is orthogonal to |θ1>A, only the first term of Eq. () contributes to the inner product in Eq. () if j = k = 1; and since the inner product of |θ1>A with itself is unity because of normalization, Eq. () reduces for j = k = 1 to
Finally, the expression on the right hand side of Eq. () is evaluated by using the law of Malus, which is preserved in the quantum mechanical treatment of polarization states: that the probability for a photon polarized in a direction n to pass through an ideal polarization analyzer with axis of transmission n′ equals the squared cosine of the angle between n and n′. Hence
where σ is b − a. Likewise,
and
The expectation value of the product of the results j and k of the polarization analyzes of photons A and B by their respective analyzers is
The required quantum mechanical predictions are thus 1/2 cos2 σ for coincidence probabilities and cos 2σ for quantum correlation
s, where σ is the angle between the detectors.
Then
and
Therefore the quantum mechanical prediction for the CHSH test statistic is
exceeding the CHSH Bell test limit of 2 and thus completing the proof of a version of Bell's Theorem. In fact, all entangled quantum states yield predictions in violation of the inequality, as Gisin (1991) and Popescu and Rohrlich (1992) have independently demonstrated. Popescu and Rohrlich (1992) also show that the maximum amount of violation is achieved with a quantum state of maximum degree of entanglement, exemplified by |Φ> of Eq. ().
Physics
Physics is a natural science that involves the study of matter and its motion through spacetime, along with related concepts such as energy and force. More broadly, it is the general analysis of nature, conducted in order to understand how the universe behaves.Physics is one of the oldest academic...
, the quantum mechanical Bell test prediction is the prediction that quantum mechanics would give for the correlation
Correlation
In statistics, dependence refers to any statistical relationship between two random variables or two sets of data. Correlation refers to any of a broad class of statistical relationships involving dependence....
probabilities for a set of measurements performed on a quantum entangled state. An important outcome of this prediction is that it violates the Bell inequality, which, as a result, has serious implications for the interpretation of quantum mechanics
Interpretation of quantum mechanics
An interpretation of quantum mechanics is a set of statements which attempt to explain how quantum mechanics informs our understanding of nature. Although quantum mechanics has held up to rigorous and thorough experimental testing, many of these experiments are open to different interpretations...
.
The following is based on section 2 of the Stanford Encyclopedia of Philosophy
Stanford Encyclopedia of Philosophy
The Stanford Encyclopedia of Philosophy is a freely-accessible online encyclopedia of philosophy maintained by Stanford University. Each entry is written and maintained by an expert in the field, including professors from over 65 academic institutions worldwide...
article written by Abner Shimony
Abner Shimony
Abner Shimony is an American physicist and philosopher of science specializing in quantum theory.-Career:Shimony obtained his BA in Mathematics and Philosophy from Yale University in 1948, and an MA in Philosophy from the University of Chicago in 1950. He obtained his Ph.D...
, one of the authors of the original Clauser, Horne, Shimony and Holt article (1969) after which the CHSH Bell test is named (Shimony, 2004).
Shimony's derivation of the quantum mechanical prediction
Let the system consist of a pair of photonPhoton
In physics, a photon is an elementary particle, the quantum of the electromagnetic interaction and the basic unit of light and all other forms of electromagnetic radiation. It is also the force carrier for the electromagnetic force...
s A and B propagating respectively in the z and −z directions. The two kets |x>j and |y>j constitute a polarization
Photon polarization
Photon polarization is the quantum mechanical description of the classical polarized sinusoidal plane electromagnetic wave. Individual photons are completely polarized...
basis for photon j (j =A, B), the former representing (in Dirac's notation) a state in which the photon A is linearly polarized in the x-direction and the latter a state in which it is linearly polarized in the y-direction. For the two-photon system the four product kets |x>A |x>B, |x>A |y>B, |y>A |x>B, and |y>A |y>B constitute a polarization basis. Each two-photon polarization state can be expressed as a linear combination of these four basis states with complex coefficients. Of particular interest are the entangled quantum states, which in no way can be expressed as |φ>A|ξ>B, with |φ> and |ξ> single-photon states, an example being
which has the useful property of being invariant under rotation of the x and y axes in the plane perpendicular to z. Neither photon A nor photon B is in a definite polarization state when the pair is in the state |Φ>, but their potentialities (in the terminology of Heisenberg 1958) are correlated: if by measurement or some other process the potentiality of photon A to be polarized along the x-direction or along the y-direction is actualized, then the same will be true of photon B, and conversely.

Crystal
A crystal or crystalline solid is a solid material whose constituent atoms, molecules, or ions are arranged in an orderly repeating pattern extending in all three spatial dimensions. The scientific study of crystals and crystal formation is known as crystallography...
polarization analyzers, with the entrance face of each analyzer perpendicular to z. Each analyzer has the property of separating light incident upon its face into two outgoing non-parallel rays, the ordinary ray and the extraordinary ray. The transmission axis of the analyzer is a direction with the property that a photon polarized along it will emerge in the ordinary ray (with certainty if the crystals are assumed to be ideal), while a photon polarized in a direction perpendicular to z and to the transmission axis will emerge in the extraordinary ray. (See diagram.)
The crystals are also idealized by assuming that no incident photon is absorbed, but each emerges in either the '+' or the '−' channel. Quantum mechanics provides an algorithm for computing the probabilities that photons A and B will emerge from these idealized analyzers in specified rays, as functions of the orientations a and b of the analyzers, a being the angle between the transmission axis of the 'A' analyzer and an arbitrary fixed direction in the x–y plane, and b having the analogous meaning for B:
Here j is a quantum number
Quantum number
Quantum numbers describe values of conserved quantities in the dynamics of the quantum system. Perhaps the most peculiar aspect of quantum mechanics is the quantization of observable quantities. This is distinguished from classical mechanics where the values can range continuously...
associated with the ray into which photon A emerges, taking values +1 or −1 depending on which channel it emerges from, while k is the analogous quantum number for photon B; and | <Φ|θj>A |ξk>B | is the ket representing the quantum state of photons A and B with the respective quantum numbers j and k. Calculation of the probabilities of interest from Eq. () can be simplified by using the invariance noted after Eq. () and rewriting |Φ> as
Eq. () results from Eq. () by substituting the transmission axis of the A analyzer for x and the direction perpendicular to both z and this transmission axis for y.
Since |θ−1>A is orthogonal to |θ1>A, only the first term of Eq. () contributes to the inner product in Eq. () if j = k = 1; and since the inner product of |θ1>A with itself is unity because of normalization, Eq. () reduces for j = k = 1 to
Finally, the expression on the right hand side of Eq. () is evaluated by using the law of Malus, which is preserved in the quantum mechanical treatment of polarization states: that the probability for a photon polarized in a direction n to pass through an ideal polarization analyzer with axis of transmission n′ equals the squared cosine of the angle between n and n′. Hence
where σ is b − a. Likewise,
and
The expectation value of the product of the results j and k of the polarization analyzes of photons A and B by their respective analyzers is
The required quantum mechanical predictions are thus 1/2 cos2 σ for coincidence probabilities and cos 2σ for quantum correlation
Quantum correlation
In Bell test experiments the term quantum correlation has come to mean the expectation value of the product of the outcomes on the two sides. In other words, the expected change in physical characteristics as one quantum system passes through an interaction site...
s, where σ is the angle between the detectors.
Demonstration of a Bell inequality violation
Now choose as the orientation angles of the transmission axesThen
and
Therefore the quantum mechanical prediction for the CHSH test statistic is
exceeding the CHSH Bell test limit of 2 and thus completing the proof of a version of Bell's Theorem. In fact, all entangled quantum states yield predictions in violation of the inequality, as Gisin (1991) and Popescu and Rohrlich (1992) have independently demonstrated. Popescu and Rohrlich (1992) also show that the maximum amount of violation is achieved with a quantum state of maximum degree of entanglement, exemplified by |Φ> of Eq. ().

