
Polignac's conjecture
Encyclopedia
In number theory
, Polignac's conjecture was made by Alphonse de Polignac
in 1849 and states:
The conjecture has not been proven or disproven for any value of n.
For n = 2, it is the twin prime conjecture. For n = 4, it says there are infinitely many cousin prime
s (p, p + 4). For n = 6, it says there are infinitely many sexy prime
s (p, p + 6) with no prime between p and p + 6.
Dickson's conjecture
generalizes Polignac's conjecture to cover all prime constellations; the Bateman–Horn conjecture gives conjectured asymptotic densities.
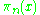 for even n be the number of prime gaps of size n below x.
for even n be the number of prime gaps of size n below x.
The first Hardy–Littlewood conjecture says the asymptotic density is of form
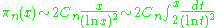
where Cn is a function of n, and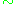 means that the quotient of two expressions tends to
means that the quotient of two expressions tends to
1 as x approaches infinity.
C2 is the twin prime constant
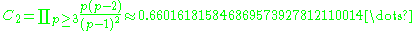
where the product extends over all prime numbers p ≥ 3.
Cn is C2 multiplied by a number which depends on the odd prime factors q of n:
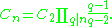
For example, C4 = C2 and C6 = 2C2. Twin primes have the same conjectured density as cousin primes, and half that of sexy primes.
Note that each odd prime factor q of n increases the conjectured density compared to twin primes by a factor of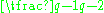 . A heuristic argument
. A heuristic argument
follows. It relies on some unproven assumptions so the conclusion remains a conjecture. The chance of a random odd prime q dividing either a or a + 2 in a random "potential" twin prime pair is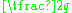 , since q divides 1 of the q numbers from a to a + q − 1. Now assume q divides n and consider a potential prime pair (a, a + n). q divides a + n if and only if q divides a, and the chance of that is
, since q divides 1 of the q numbers from a to a + q − 1. Now assume q divides n and consider a potential prime pair (a, a + n). q divides a + n if and only if q divides a, and the chance of that is 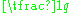 . The chance of (a, a + n) being free from the factor q, divided by the chance that (a, a + 2) is free from q, then becomes
. The chance of (a, a + n) being free from the factor q, divided by the chance that (a, a + 2) is free from q, then becomes  divided by
divided by 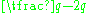 . This equals
. This equals 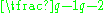 which transfers to the conjectured prime density. In the case of n = 6, the argument simplifies to: If a is a random number then 3 has chance 2/3 of dividing a or a + 2, but only chance 1/3 of dividing a and a + 6, so the latter pair is conjectured twice as likely to both be prime.
which transfers to the conjectured prime density. In the case of n = 6, the argument simplifies to: If a is a random number then 3 has chance 2/3 of dividing a or a + 2, but only chance 1/3 of dividing a and a + 6, so the latter pair is conjectured twice as likely to both be prime.
Number theory
Number theory is a branch of pure mathematics devoted primarily to the study of the integers. Number theorists study prime numbers as well...
, Polignac's conjecture was made by Alphonse de Polignac
Alphonse de Polignac
Alphonse de Polignac was a French mathematician. In 1849 he made Polignac's conjecture:The case k = 1 is the twin prime conjecture.-References:...
in 1849 and states:
- For any positive even number n, there are infinitely many prime gaps of size n. In other words: There are infinitely many cases of two consecutive prime numberPrime numberA prime number is a natural number greater than 1 that has no positive divisors other than 1 and itself. A natural number greater than 1 that is not a prime number is called a composite number. For example 5 is prime, as only 1 and 5 divide it, whereas 6 is composite, since it has the divisors 2...
s with difference n.
The conjecture has not been proven or disproven for any value of n.
For n = 2, it is the twin prime conjecture. For n = 4, it says there are infinitely many cousin prime
Cousin prime
In mathematics, cousin primes are prime numbers that differ by four; compare this with twin primes, pairs of prime numbers that differ by two, and sexy primes, pairs of prime numbers that differ by six....
s (p, p + 4). For n = 6, it says there are infinitely many sexy prime
Sexy prime
In mathematics, a sexy prime is a prime number that differs from another prime number by six. For example, the numbers 5 and 11 are both sexy primes, because they differ by 6...
s (p, p + 6) with no prime between p and p + 6.
Dickson's conjecture
Dickson's conjecture
In number theory, a branch of mathematics, Dickson's conjecture is the conjecture stated by that for a finite set of linear forms , , ..., with , there are infinitely many positive integers for which they are all prime, unless there is a congruence condition preventing this...
generalizes Polignac's conjecture to cover all prime constellations; the Bateman–Horn conjecture gives conjectured asymptotic densities.
Conjectured density
Let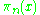 for even n be the number of prime gaps of size n below x.
for even n be the number of prime gaps of size n below x.The first Hardy–Littlewood conjecture says the asymptotic density is of form
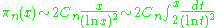
where Cn is a function of n, and
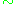 means that the quotient of two expressions tends to
means that the quotient of two expressions tends toLimit (mathematics)
In mathematics, the concept of a "limit" is used to describe the value that a function or sequence "approaches" as the input or index approaches some value. The concept of limit allows mathematicians to define a new point from a Cauchy sequence of previously defined points within a complete metric...
1 as x approaches infinity.
C2 is the twin prime constant
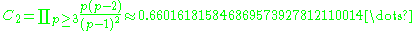
where the product extends over all prime numbers p ≥ 3.
Cn is C2 multiplied by a number which depends on the odd prime factors q of n:
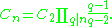
For example, C4 = C2 and C6 = 2C2. Twin primes have the same conjectured density as cousin primes, and half that of sexy primes.
Note that each odd prime factor q of n increases the conjectured density compared to twin primes by a factor of
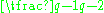 . A heuristic argument
. A heuristic argumentHeuristic argument
A heuristic argument is an argument that reasons from the value of a method or principle that has been shown by experimental investigation to be a useful aid in learning, discovery and problem-solving. A widely-used and important example of a heuristic argument is Occam's Razor....
follows. It relies on some unproven assumptions so the conclusion remains a conjecture. The chance of a random odd prime q dividing either a or a + 2 in a random "potential" twin prime pair is
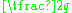 , since q divides 1 of the q numbers from a to a + q − 1. Now assume q divides n and consider a potential prime pair (a, a + n). q divides a + n if and only if q divides a, and the chance of that is
, since q divides 1 of the q numbers from a to a + q − 1. Now assume q divides n and consider a potential prime pair (a, a + n). q divides a + n if and only if q divides a, and the chance of that is 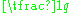 . The chance of (a, a + n) being free from the factor q, divided by the chance that (a, a + 2) is free from q, then becomes
. The chance of (a, a + n) being free from the factor q, divided by the chance that (a, a + 2) is free from q, then becomes  divided by
divided by 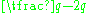 . This equals
. This equals 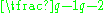 which transfers to the conjectured prime density. In the case of n = 6, the argument simplifies to: If a is a random number then 3 has chance 2/3 of dividing a or a + 2, but only chance 1/3 of dividing a and a + 6, so the latter pair is conjectured twice as likely to both be prime.
which transfers to the conjectured prime density. In the case of n = 6, the argument simplifies to: If a is a random number then 3 has chance 2/3 of dividing a or a + 2, but only chance 1/3 of dividing a and a + 6, so the latter pair is conjectured twice as likely to both be prime.

