Pappus's hexagon theorem
Encyclopedia
Pappus of Alexandria
Pappus of Alexandria was one of the last great Greek mathematicians of Antiquity, known for his Synagoge or Collection , and for Pappus's Theorem in projective geometry...
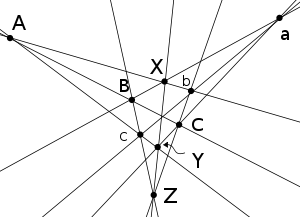
) states that given one set of collinear
Line (geometry)
The notion of line or straight line was introduced by the ancient mathematicians to represent straight objects with negligible width and depth. Lines are an idealization of such objects...
points A, B, C, and another set of collinear points a, b, c, then the intersection points X, Y, Z of line
Line (mathematics)
The notion of line or straight line was introduced by the ancient mathematicians to represent straight objects with negligible width and depth. Lines are an idealization of such objects...
pairs Ab and aB, Ac and aC, Bc and bC are collinear. (Collinear means the points are incident on a line.) It holds in the projective plane
Projective plane
In mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect in a single point, but there are some pairs of lines that do not intersect...
over any field, but fails for the projective plane over any non-commutative division ring
Division ring
In abstract algebra, a division ring, also called a skew field, is a ring in which division is possible. Specifically, it is a non-trivial ring in which every non-zero element a has a multiplicative inverse, i.e., an element x with...
.
The dual
Duality (projective geometry)
A striking feature of projective planes is the "symmetry" of the roles played by points and lines in the definitions and theorems, and duality is the formalization of this metamathematical concept. There are two approaches to the subject of duality, one through language and the other a more...
of this theorem
Theorem
In mathematics, a theorem is a statement that has been proven on the basis of previously established statements, such as other theorems, and previously accepted statements, such as axioms...
states that given one set of concurrent lines
Concurrent lines
In geometry, two or more lines are said to be concurrent if they intersect at a single point.In a triangle, four basic types of sets of concurrent lines are altitudes, angle bisectors, medians, and perpendicular bisectors:...
A, B, C, and another set of concurrent lines a, b, c, then the lines x, y, z defined by pairs of points resulting from pairs of intersections A∩b and a∩B, A∩c and a∩C, B∩c and b∩C are concurrent. (Concurrent means that the lines pass through one point.)
Pappus's theorem is a special case of Pascal's theorem
Pascal's theorem
In projective geometry, Pascal's theorem states that if an arbitrary hexagon is inscribed in any conic section, and pairs of opposite sides are extended until they meet, the three intersection points will lie on a straight line, the Pascal line of that configuration.- Related results :This theorem...
for a conic, in the limiting case when the conic degenerates
Degenerate conic
In mathematics, a degenerate conic is a conic that fails to be an irreducible curve...
into 2 straight lines.
The Pappus configuration is the configuration of 9 lines and 9 points that occurs in Pappus's theorem, with each line meeting 3 of the points and each point meeting 3 lines. This configuration is self dual
Duality (projective geometry)
A striking feature of projective planes is the "symmetry" of the roles played by points and lines in the definitions and theorems, and duality is the formalization of this metamathematical concept. There are two approaches to the subject of duality, one through language and the other a more...
.
The Levi graph
Levi graph
In combinatorial mathematics, a Levi graph or incidence graph is a bipartite graph associated with an incidence structure. From a collection of points and lines in an incidence geometry or a projective configuration, we form a graph with one vertex per point, one vertex per line, and an edge for...
of the Pappus configuration is the Pappus graph
Pappus graph
In the mathematical field of graph theory, the Pappus graph is a 3-regular undirected graph with 18 vertices and 27 edges, formed as the Levi graph of the Pappus configuration. It is named after Pappus of Alexandria, an ancient Greek mathematician who is believed to have discovered the "hexagon...
, a bipartite
Bipartite graph
In the mathematical field of graph theory, a bipartite graph is a graph whose vertices can be divided into two disjoint sets U and V such that every edge connects a vertex in U to one in V; that is, U and V are independent sets...
distance-regular
Distance-regular graph
In mathematics, a distance-regular graph is a regular graph such that for any two vertices v and w at distance i the number of vertices adjacent to w and at distance j from v is the same. Every distance-transitive graph is distance-regular...
graph with 18 vertices and 27 edges.
Proof
Pappus's theorem is equivalent to its dual (in the presence of the basic axioms for a projective plane).The dual of Pappus's theorem can be proved for the projective plane over any field using projective coordinates as follows.
The dual of the theorem states that if all but one of the nine sets of three lines are concurrent, then all of them are.
Choose projective coordinates with
- C=(1,0,0), c=(0,1,0), X=(0,0,1), A=(1,1,1).
On the lines AC, Ac, AX, given by x2=x3, x1=x3, x2=x1, take the points B, b, Y to be
- B=(p,1,1), b=(1,q,1), Y=(1,1,r)
for some p, q, r. The three lines XB, cb, CY are x1=px2, x2=qx3, x3=rx1, so all pass through the same point (called a in the diagram) if and only if pqr=1. The condition for the three lines Cb, cB and XY x2=qx1, x1=px3, x3=rx2 to pass through the same point (Z in the diagram) is qpr=1. So this last set of three lines is concurrent if all the other eight sets are because multiplication is commutative, so pqr=qpr, which proves the dual of Pappus's theorem.
The proof above also shows that if Pappus's theorem holds for a projective space over a division ring, then the division ring is a (commutative) field. German mathematician Gerhard Hessenberg
Gerhard Hessenberg
Gerhard Hessenberg was a German mathematician. He received his Ph.D from the University of Berlin in 1899 under the guidance of Hermann Schwarz and Lazarus Fuchs...
proved that Pappus's theorem implies Desargues's theorem. In general, Pappus's theorem holds for some projective space if and only if it is a projective space over a commutative field. The projective spaces in which Pappus's theorem does not hold are projective spaces over non-commutative division rings, and non-Desarguesian plane
Non-Desarguesian plane
In mathematics, a non-Desarguesian plane, named after Gérard Desargues, is a projective plane that does not satisfy Desargues's theorem, or in other words a plane that is not a Desarguesian plane...
s.
Other Statements of Pappus's Theorem
In addition to the above characterizations of Pappus's Theorem and its dual, the following are equivalent statements:- If the six vertices of a hexagon lie alternately on two lines, then the three points of intersection of pairs of opposite sides are collinear.
-
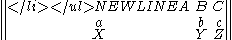In a matrix of 9 points (as in the picture and description above), if the first two rows and the six "diagonal" triads are collinear, then the third row is collinear. That is, if ABC, abc, AbZ, BcX, CaY, XbC, YcA, and ZaB are all lines, then Pappus's theorem states that XYZ must be a line. Also, note that the same matrix formulation applys when A, B, C and a, b, c are concurrent lines in the dual form of the theorem.
- Given three distinct points on each of two distinct lines, pair each point on one of the lines with one from the other line, then the joins of points not paired will meet in (opposite) pairs at points along a line.
- If two triangles are doubly perspectivePerspective (geometry)In geometry, two triangles are perspective if, when the sides of each triangle are extended, they meet at three collinear points. The line which goes through the three points is known as the perspectrix, perspective axis, homology axis, or axis of perspectivity. The triangles are said to be...
, then they are trebly perspective. - If AB, CD, and EF are concurrent and DE, FA, and BC are concurrent, then AD, BE, and CF are concurrent.
External links
- Pappus' hexagon theorem at cut-the-knotCut-the-knotCut-the-knot is a free, advertisement-funded educational website maintained by Alexander Bogomolny and devoted to popular exposition of many topics in mathematics. The site has won more than 20 awards from scientific and educational publications, including a Scientific American Web Award in 2003,...
- Dual to Pappus' hexagon theorem at cut-the-knotCut-the-knotCut-the-knot is a free, advertisement-funded educational website maintained by Alexander Bogomolny and devoted to popular exposition of many topics in mathematics. The site has won more than 20 awards from scientific and educational publications, including a Scientific American Web Award in 2003,...