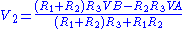Nodal analysis
Encyclopedia
In electric circuits analysis, nodal analysis, node-voltage analysis, or the branch current method is a method of determining the voltage (potential difference) between "nodes
" (points where elements or branches connect) in an electrical circuit in terms of the branch currents.
In analyzing a circuit using Kirchhoff's circuit laws
, one can either do nodal analysis using Kirchhoff's current law (KCL) or mesh analysis
using Kirchhoff's voltage law (KVL). Nodal analysis writes an equation at each electrical node
, requiring that the branch currents incident at a node must sum to zero. The branch currents are written in terms of the circuit node voltages. As a consequence, each branch constitutive relation must give current as a function of voltage; an admittance
representation. For instance, for a resistor, Ibranch = Vbranch * G, where G (=1/R) is the admittance (conductance) of the resistor.
Nodal analysis is possible when all the circuit elements' branch constitutive relations have an admittance representation. Nodal analysis produces a compact set of equations for the network, which can be solved by hand if small, or can be quickly solved using linear algebra by computer. Because of the compact system of equations, many circuit simulation programs (e.g. SPICE
) use nodal analysis as a basis. When elements do not have admittance representations, a more general extension of nodal analysis, modified nodal analysis
, can be used.
While simple examples of nodal analysis focus on linear elements, more complex nonlinear networks can also be solved with nodal analysis by using Newton's method
to turn the nonlinear problem into a sequence of linear problems.
With Kirchhoff's current law, we get:
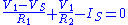
This equation can be solved in respect to V1:
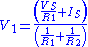
Finally, the unknown voltage can be solved by substituting numerical values for the symbols. Any unknown currents are easy to calculate after all the voltages in the circuit are known.
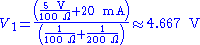
The current going through voltage source VA cannot be directly calculated. Therefore we can not write the current equations for either V1 or V2. However, we know that the same current leaving node V2 must enter node V1. Even though the nodes can not be individually solved, we know that the combined current of these two nodes is zero. This combining of the two nodes is called the supernode
technique, and it requires one additional equation: V1 = V2 + VA.
The complete set of equations for this circuit is:
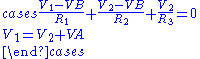
By substituting V1 to the first equation and solving in respect to V2, we get:
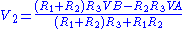
Node (circuits)
In electrical engineering, node refers to any point on a circuit where two or more circuit elements meet. For two nodes to be different, their voltages must be different. Without any further knowledge, it is easy to establish how to find a node by using Ohm's Law: V=IR. When looking at circuit...
" (points where elements or branches connect) in an electrical circuit in terms of the branch currents.
In analyzing a circuit using Kirchhoff's circuit laws
Kirchhoff's circuit laws
Kirchhoff's circuit laws are two equalities that deal with the conservation of charge and energy in electrical circuits, and were first described in 1845 by Gustav Kirchhoff...
, one can either do nodal analysis using Kirchhoff's current law (KCL) or mesh analysis
Mesh analysis
Mesh analysis is a method that is used to solve planar circuits for the currents at any place in the circuit. Planar circuits are circuits that can be drawn on a plane surface with no wires crossing each other. A more general technique, called loop analysis can be applied to any circuit, planar...
using Kirchhoff's voltage law (KVL). Nodal analysis writes an equation at each electrical node
Node (circuits)
In electrical engineering, node refers to any point on a circuit where two or more circuit elements meet. For two nodes to be different, their voltages must be different. Without any further knowledge, it is easy to establish how to find a node by using Ohm's Law: V=IR. When looking at circuit...
, requiring that the branch currents incident at a node must sum to zero. The branch currents are written in terms of the circuit node voltages. As a consequence, each branch constitutive relation must give current as a function of voltage; an admittance
Admittance
In electrical engineering, the admittance is a measure of how easily a circuit or device will allow a current to flow. It is defined as the inverse of the impedance . The SI unit of admittance is the siemens...
representation. For instance, for a resistor, Ibranch = Vbranch * G, where G (=1/R) is the admittance (conductance) of the resistor.
Nodal analysis is possible when all the circuit elements' branch constitutive relations have an admittance representation. Nodal analysis produces a compact set of equations for the network, which can be solved by hand if small, or can be quickly solved using linear algebra by computer. Because of the compact system of equations, many circuit simulation programs (e.g. SPICE
SPICE
SPICE is a general-purpose, open source analog electronic circuit simulator.It is a powerful program that is used in integrated circuit and board-level design to check the integrity of circuit designs and to predict circuit behavior.- Introduction :Unlike board-level designs composed of discrete...
) use nodal analysis as a basis. When elements do not have admittance representations, a more general extension of nodal analysis, modified nodal analysis
Modified nodal analysis
In Electrical Engineering Modified Nodal Analysis or MNA is an extension of nodal analysis which not only determines the circuit's node voltages , but also some branch currents. Modified nodal analysis was developed as a formalism to mitigate the difficulty of representing voltage-defined...
, can be used.
While simple examples of nodal analysis focus on linear elements, more complex nonlinear networks can also be solved with nodal analysis by using Newton's method
Newton's method
In numerical analysis, Newton's method , named after Isaac Newton and Joseph Raphson, is a method for finding successively better approximations to the roots of a real-valued function. The algorithm is first in the class of Householder's methods, succeeded by Halley's method...
to turn the nonlinear problem into a sequence of linear problems.
Method
- Note all connected wire segments in the circuit. These are the nodes of nodal analysis.
- Select one node as the ground reference. The choice does not affect the result and is just a matter of convention. Choosing the node with most connections can simplify the analysis.
- Assign a variable for each node whose voltage is unknown. If the voltage is already known, it is not necessary to assign a variable.
- For each unknown voltage, form an equation based on Kirchhoff's current law. Basically, add together all currents leaving from the node and mark the sum equal to zero.
- If there are voltage sources between two unknown voltages, join the two nodes as a supernodeSupernode (circuit)In circuit theory, a super-node is a theoretical construct that can be used to solve a circuit. This is done by viewing a voltage source on a wire as a point source voltage in relation to other point voltages located at various nodes in the circuit, relative to a ground node assigned a zero or...
. The currents of the two nodes are combined in a single equation, and a new equation for the voltages is formed. - Solve the system of simultaneous equationsSimultaneous equationsIn mathematics, simultaneous equations are a set of equations containing multiple variables. This set is often referred to as a system of equations. A solution to a system of equations is a particular specification of the values of all variables that simultaneously satisfies all of the equations...
for each unknown voltage.
Basic case
The only unknown voltage in this circuit is V1. There are three connections to this node and consequently three currents to consider. The direction of the currents in calculations is chosen to be away from the node.- Current through resistor R1: (V1 - VS) / R1
- Current through resistor R2: V1 / R2
- Current through current source IS: -IS
With Kirchhoff's current law, we get:
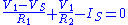
This equation can be solved in respect to V1:
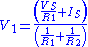
Finally, the unknown voltage can be solved by substituting numerical values for the symbols. Any unknown currents are easy to calculate after all the voltages in the circuit are known.
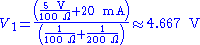
Supernodes
In this circuit, we initially have two unknown voltages, V1 and V2. The voltage at V3 is already known to be VB because the other terminal of the voltage source is at ground potential.The current going through voltage source VA cannot be directly calculated. Therefore we can not write the current equations for either V1 or V2. However, we know that the same current leaving node V2 must enter node V1. Even though the nodes can not be individually solved, we know that the combined current of these two nodes is zero. This combining of the two nodes is called the supernode
Supernode (circuit)
In circuit theory, a super-node is a theoretical construct that can be used to solve a circuit. This is done by viewing a voltage source on a wire as a point source voltage in relation to other point voltages located at various nodes in the circuit, relative to a ground node assigned a zero or...
technique, and it requires one additional equation: V1 = V2 + VA.
The complete set of equations for this circuit is:
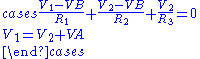
By substituting V1 to the first equation and solving in respect to V2, we get: