
Neutrino theory of light
Encyclopedia
The neutrino theory of light is the proposal that the photon
is a composite particle formed of a neutrino
-antineutrino pair
. It is based on the idea that emission and absorption
of a photon corresponds to the creation and annihilation of a particle-antiparticle pair. The neutrino
theory of light is not currently accepted as part of mainstream physics, as according to the standard model
the photon is an elementary particle
, a gauge boson
.
Historically, many particles that were once thought to be elementary such as protons, neutrons, pions, and kaons have turned out to be composite particles. In 1932, Louis de Broglie suggested that the photon might be the combination of a neutrino and an antineutrino. During the 1930s there was great interest in the neutrino theory of light and Pascual Jordan
, Ralph Kronig
, Max Born
, and others worked on the theory.
In 1938, Maurice Henry Lecorney Pryce brought work on the composite photon theory to halt. He showed that the conditions imposed by Bose-Einstein commutation relations for the composite photon and the connection between its spin and polarization were incompatible. Pryce also pointed out other possible problems, “In so far as the failure of the theory can be traced to any one cause it is fair to say that it lies in the fact that light waves are polarized transversely while neutrino ‘waves’ are polarized longitudinally,” and lack of rotational invariance. In 1966, V S Berezinskii reanalyzed Pryce’s paper, giving a clearer picture of the problem that Pryce uncovered.
Starting in the 1960s work on the neutrino theory of light resumed, and there continues to be some interest in recent years. Attempts have been made to solve the problem pointed out by Pryce, known as Pryce’s Theorem, and other problems with the composite photon theory. The incentive is seeing the natural way that many photon properties are generated from the theory and the knowledge that some problems exist with the current photon model. However, there is no experimental evidence that the photon has a composite structure.
Some of the problems for the neutrino theory of light are the non-existence for massless neutrinos with both spin parallel and antiparallel to their momentum and the fact that composite photons are not bosons. Attempts to solve some of these problems will be discussed, but the lack of massless neutrinos makes it impossible to form a massless photon with this theory. The neutrino theory of light is not considered to be part of mainstream
physics.
photons from neutrinos.
with the mass set to zero,
Photon
In physics, a photon is an elementary particle, the quantum of the electromagnetic interaction and the basic unit of light and all other forms of electromagnetic radiation. It is also the force carrier for the electromagnetic force...
is a composite particle formed of a neutrino
Neutrino
A neutrino is an electrically neutral, weakly interacting elementary subatomic particle with a half-integer spin, chirality and a disputed but small non-zero mass. It is able to pass through ordinary matter almost unaffected...
-antineutrino pair
Pair production
Pair production refers to the creation of an elementary particle and its antiparticle, usually from a photon . For example an electron and its antiparticle, the positron, may be created...
. It is based on the idea that emission and absorption
Absorption (electromagnetic radiation)
In physics, absorption of electromagnetic radiation is the way by which the energy of a photon is taken up by matter, typically the electrons of an atom. Thus, the electromagnetic energy is transformed to other forms of energy for example, to heat. The absorption of light during wave propagation is...
of a photon corresponds to the creation and annihilation of a particle-antiparticle pair. The neutrino
Neutrino
A neutrino is an electrically neutral, weakly interacting elementary subatomic particle with a half-integer spin, chirality and a disputed but small non-zero mass. It is able to pass through ordinary matter almost unaffected...
theory of light is not currently accepted as part of mainstream physics, as according to the standard model
Standard Model
The Standard Model of particle physics is a theory concerning the electromagnetic, weak, and strong nuclear interactions, which mediate the dynamics of the known subatomic particles. Developed throughout the mid to late 20th century, the current formulation was finalized in the mid 1970s upon...
the photon is an elementary particle
Elementary particle
In particle physics, an elementary particle or fundamental particle is a particle not known to have substructure; that is, it is not known to be made up of smaller particles. If an elementary particle truly has no substructure, then it is one of the basic building blocks of the universe from which...
, a gauge boson
Gauge boson
In particle physics, gauge bosons are bosonic particles that act as carriers of the fundamental forces of nature. More specifically, elementary particles whose interactions are described by gauge theory exert forces on each other by the exchange of gauge bosons, usually as virtual particles.-...
.
Historically, many particles that were once thought to be elementary such as protons, neutrons, pions, and kaons have turned out to be composite particles. In 1932, Louis de Broglie suggested that the photon might be the combination of a neutrino and an antineutrino. During the 1930s there was great interest in the neutrino theory of light and Pascual Jordan
Pascual Jordan
-Further reading:...
, Ralph Kronig
Ralph Kronig
Ralph Kronig was a German-American physicist . He is noted for the discovery of particle spin and for his theory of x-ray absorption spectroscopy...
, Max Born
Max Born
Max Born was a German-born physicist and mathematician who was instrumental in the development of quantum mechanics. He also made contributions to solid-state physics and optics and supervised the work of a number of notable physicists in the 1920s and 30s...
, and others worked on the theory.
In 1938, Maurice Henry Lecorney Pryce brought work on the composite photon theory to halt. He showed that the conditions imposed by Bose-Einstein commutation relations for the composite photon and the connection between its spin and polarization were incompatible. Pryce also pointed out other possible problems, “In so far as the failure of the theory can be traced to any one cause it is fair to say that it lies in the fact that light waves are polarized transversely while neutrino ‘waves’ are polarized longitudinally,” and lack of rotational invariance. In 1966, V S Berezinskii reanalyzed Pryce’s paper, giving a clearer picture of the problem that Pryce uncovered.
Starting in the 1960s work on the neutrino theory of light resumed, and there continues to be some interest in recent years. Attempts have been made to solve the problem pointed out by Pryce, known as Pryce’s Theorem, and other problems with the composite photon theory. The incentive is seeing the natural way that many photon properties are generated from the theory and the knowledge that some problems exist with the current photon model. However, there is no experimental evidence that the photon has a composite structure.
Some of the problems for the neutrino theory of light are the non-existence for massless neutrinos with both spin parallel and antiparallel to their momentum and the fact that composite photons are not bosons. Attempts to solve some of these problems will be discussed, but the lack of massless neutrinos makes it impossible to form a massless photon with this theory. The neutrino theory of light is not considered to be part of mainstream
Standard Model
The Standard Model of particle physics is a theory concerning the electromagnetic, weak, and strong nuclear interactions, which mediate the dynamics of the known subatomic particles. Developed throughout the mid to late 20th century, the current formulation was finalized in the mid 1970s upon...
physics.
Forming photon from neutrinos
Actually, it is not difficult to obtain transversely polarizedphotons from neutrinos.
The neutrino field
The neutrino field satisfies the Dirac equationDirac equation
The Dirac equation is a relativistic quantum mechanical wave equation formulated by British physicist Paul Dirac in 1928. It provided a description of elementary spin-½ particles, such as electrons, consistent with both the principles of quantum mechanics and the theory of special relativity, and...
with the mass set to zero,
-
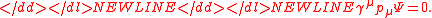
The gamma matrices in the Weyl basis are:-

-

The matrix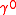 is Hermitian while
is Hermitian while 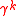 is antihermitian. They satisfy the anticommutation relation,
is antihermitian. They satisfy the anticommutation relation,
-
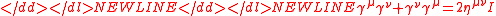
where is the Minkowski metric with signature
is the Minkowski metric with signature  and
and 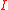 is the unit matrix.
is the unit matrix.
The neutrino field is given by,-

-

where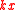 stands for
stands for  .
.
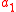 and
and 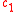 are the fermion annihilation operators for
are the fermion annihilation operators for 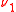
and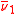 respectively, while
respectively, while 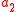 and
and 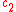 are
are
the annihilation operators for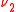 and
and 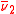 .
.
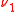 is a right-handed neutrino and
is a right-handed neutrino and  is a left-handed neutrino.
is a left-handed neutrino.
The 's are spinors with the superscripts and subscripts referring to the energy and helicity states respectively. SpinorSpinorIn mathematics and physics, in particular in the theory of the orthogonal groups , spinors are elements of a complex vector space introduced to expand the notion of spatial vector. Unlike tensors, the space of spinors cannot be built up in a unique and natural way from spatial vectors...
's are spinors with the superscripts and subscripts referring to the energy and helicity states respectively. SpinorSpinorIn mathematics and physics, in particular in the theory of the orthogonal groups , spinors are elements of a complex vector space introduced to expand the notion of spatial vector. Unlike tensors, the space of spinors cannot be built up in a unique and natural way from spatial vectors...
solutions for the Dirac equationDirac equationThe Dirac equation is a relativistic quantum mechanical wave equation formulated by British physicist Paul Dirac in 1928. It provided a description of elementary spin-½ particles, such as electrons, consistent with both the principles of quantum mechanics and the theory of special relativity, and...
are,
-

-

-

-

The neutrino spinors for negative momenta are related to those of positive momenta by,
-

-

-
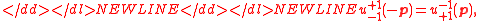
-
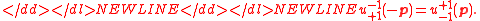
The composite photon field
De Broglie and Kronig suggested the use of a local interaction to bind the neutrino-antineutrino pair. (Rosen and Singer
have used a delta-function interaction in forming a
composite photon.)
Fermi and Yang
used a local interaction to bind
a fermion-antiferminon pair in attempting to form a pion. A four-vector field can be created from a fermion-antifermion pair,-
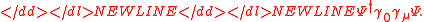
Forming the photon field can be done simply by,-
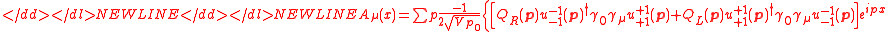
-
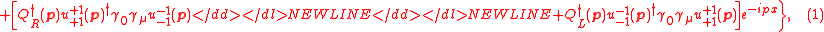
where .
.
The annihilation operators for right-handed and left-handed photons formed of fermion-antifermion pairs are defined as,-

-
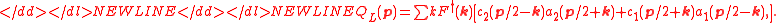
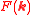 is a spectral function, normalized by
is a spectral function, normalized by
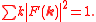
Photon polarization vectors
The polarization vectors corresponding to the combinations used
in Eq. (1) are,-

-

Carrying out the matrix multiplications results in,-
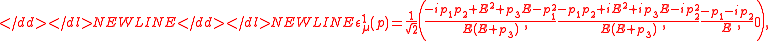
-
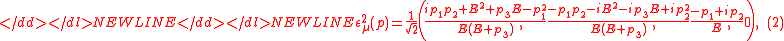
where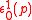 and
and  have been placed on the right.
have been placed on the right.
For massless fermions the polarization vectors depend only upon the direction of
 . Let
. Let 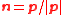 .
.
-

-

These polarization vectors satisfy the
normalization relation,-

-
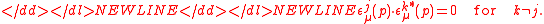
The Lorentz-invariant dot
products of the internal four-momentum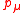
with the polarization vectors are,-

-
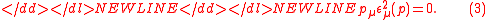
In three dimensions,-
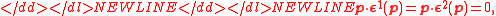
-

-

-

Composite photon satisfies Maxwell’s equations
In terms of the polarization vectors,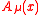 becomes,
becomes,
-
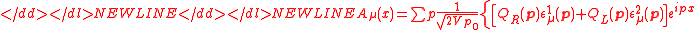
-
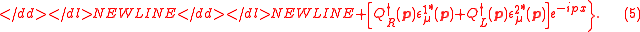
The electric field and magnetic field
and magnetic field
 are given by,
are given by,
-

-
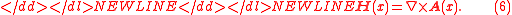
Applying Eq. (6) to Eq. (5), results in,-

-
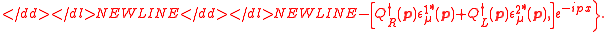
-

-
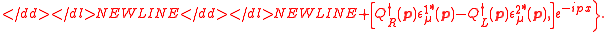
Maxwell's equationsMaxwell's equationsMaxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
for free space are obtained as follows:-
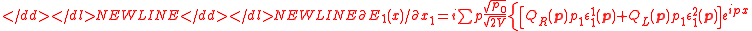
-
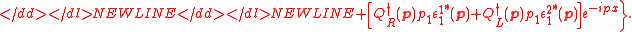
Thus,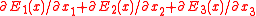 contains terms of the form
contains terms of the form
 which equate to zero by the first of Eq. (4).
which equate to zero by the first of Eq. (4).
This gives,-
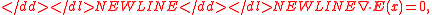
-

as contains similar terms.
contains similar terms.
The expression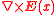 contains terms of the form
contains terms of the form
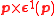 while
while

contains terms of form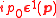 . Thus, the last two equations of (4) can be used to show that,
. Thus, the last two equations of (4) can be used to show that,
-

-

Although the neutrino field violates parityParity (physics)In physics, a parity transformation is the flip in the sign of one spatial coordinate. In three dimensions, it is also commonly described by the simultaneous flip in the sign of all three spatial coordinates:...
and charge
conjugation
,
 and
and
 transform in the usual way
transform in the usual way
,-

-
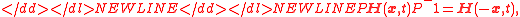
-
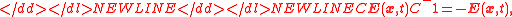
-

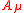 satisfies the Lorentz condition,
satisfies the Lorentz condition,
-

which follows from Eq. (3).
Although many choices for gamma matrices can satisfy the Dirac equationDirac equationThe Dirac equation is a relativistic quantum mechanical wave equation formulated by British physicist Paul Dirac in 1928. It provided a description of elementary spin-½ particles, such as electrons, consistent with both the principles of quantum mechanics and the theory of special relativity, and...
, it
is essential that one use the Weyl representation in order to get the correct photon polarization vectors and and
and  that satisfy Maxwell's equationsMaxwell's equationsMaxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
that satisfy Maxwell's equationsMaxwell's equationsMaxwell's equations are a set of partial differential equations that, together with the Lorentz force law, form the foundation of classical electrodynamics, classical optics, and electric circuits. These fields in turn underlie modern electrical and communications technologies.Maxwell's equations...
. Kronig
first realized this. In the Weyl representation,
the four-component spinors are describing two sets of two-component neutrinos.
The connection between the photon antisymmetric tensor and the two-component Weyl equation was also noted by Sen.
One can also produce the above results using a two-component neutrino theory.
To compute the commutation relations for the photon field,
one needs the equation,
-

To obtain this equation, Kronig
wrote a relation between the neutrino spinors that was not
rotationally invariant as pointed out by Pryce.
However, as Perkins showed, this equation
follows directly from summing over the polarization vectors,
Eq. (2), that were obtained by
explicitly solving for the neutrino spinors.
If the momentum is along the third axis,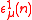
and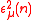 reduce to the usual polarization vectors
reduce to the usual polarization vectors
for right and left circularly polarized photons respectively.-

-
Problems with the neutrino theory of light
Although composite photons satisfy many properties of real photons,
there are major problems with this theory.
Bose–Einstein commutation relations
It is known that a photon is a boson.
Does the composite photon satisfy Bose–Einstein commutation relations? Fermions are defined as the particles whose creation and annihilation operators adhere to the anticommutation relations
-

-

-
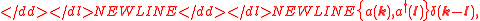
while bosons are defined as the particles that adhere to the commutation relations
-

-

-
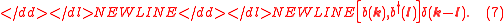
The creation and annihilation operators of composite particles formed of fermion pairs adhere to the commutation relations of the form
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-
-

