
Lifting-line theory
Encyclopedia
Lifting-line theory or Lanchester-Prandtl wing theory was published by Ludwig Prandtl in 1918–1919 after working with Albert Betz
and Max Munk on the problem of a useful mathematical tool for examining lift from "real world" wings.
In this model, the vortex strength reduces along the wingspan, and the loss in vortex strength is shed as a vortex-sheet from the trailing edge, rather than just at the wing-tips.
The following are functions of the wings span-wise station (i.e. can vary along the wing)
To derive the model we start with the assumption that the circulation of the wing varies as a function of the spanwise locations. The function assumed is a Fourier function. Firstly, the coordinate for the spanwise location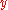 is transformed by
is transformed by 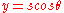 , where y is spanwise location, and s is the semi-span of the wing.
, where y is spanwise location, and s is the semi-span of the wing.
and so the circulation is assumed to be:
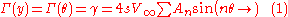
Since the circulation of a section is related the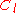 by the equation:
by the equation:
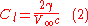
but since the coefficient of lift is a function of angle of attack:
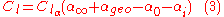
hence the vortex strength at any particular spanwise station can be given by the equations:
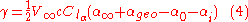
This one equation has two unknowns: the value for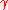 and the value for
and the value for 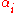 . However, the downwash is purely a function of the circulation only. So we can determine the value
. However, the downwash is purely a function of the circulation only. So we can determine the value 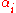 in terms of
in terms of 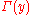 , bring this term across to the left hand side of the equation and solve. The downwash at any given station is a function of the entire shed vortex system. This is determined by integrating the influence of each differential shed vortex over the span of the wing.
, bring this term across to the left hand side of the equation and solve. The downwash at any given station is a function of the entire shed vortex system. This is determined by integrating the influence of each differential shed vortex over the span of the wing.
Differential element of circulation:
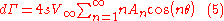
Differential downwash due to the differential element of circulation (acts like half an infinite vortex line):
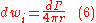
The integral equation over the span of the wing to determine the downwash at a particular location is:
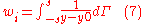
After appropriate substitutions and integrations we get:
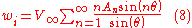
And so the change in angle attack is determined by (assuming small angles):
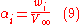
By substituting equations 8 and 9 into RHS of equation 4 and equation 1 into the LHS of equation 4, we then get:
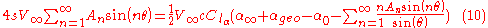
After rearranging, we get the series of simultaneous equations:


By taking a finite number of terms, equation 11 can be expressed in matrix form and solved for coefficients A. Note the left-hand side of the equation represents each element in the matrix, and the terms on the RHS of equation 11 represent the RHS of the matrix form. Each row in the matrix form represents a different span-wise station, and each column represents a different value for n.
Appropriate choices for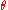 are as a linear variation between
are as a linear variation between  . Note that this range does not include the values for
. Note that this range does not include the values for  , as this will lead to a singular matrix, which can't be solved.
, as this will lead to a singular matrix, which can't be solved.
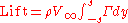
which can be reduced to:

The induced drag can be determined from
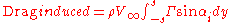
or
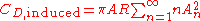


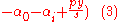
where
Note that y can be negative. This addition will introduce non-zero even coefficients in the equation which will have to accounted for.
 term in Equation 3. For non-symmetric controls such as ailerons the
term in Equation 3. For non-symmetric controls such as ailerons the  term will change on each side of the wing.
term will change on each side of the wing.

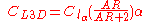
where
The theoretical value for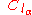 is 2
is 2 . Note that this equation becomes the thin airfoil equation if AR goes to infinity.
. Note that this equation becomes the thin airfoil equation if AR goes to infinity.
Lifting-line theory also states an equation for induced drag:.
where
Albert Betz
Albert Betz was a German physicist and a pioneer of wind turbine technology.In 1910 he graduated as a naval engineer from Technische Hochschule Berlin...
and Max Munk on the problem of a useful mathematical tool for examining lift from "real world" wings.
In this model, the vortex strength reduces along the wingspan, and the loss in vortex strength is shed as a vortex-sheet from the trailing edge, rather than just at the wing-tips.
Derivation
Nomenclature: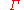 is the 3D circulation function over the wing
is the 3D circulation function over the wing is the 2D lift coefficientLift coefficientThe lift coefficient is a dimensionless coefficient that relates the lift generated by a lifting body, the dynamic pressure of the fluid flow around the body, and a reference area associated with the body...
is the 2D lift coefficientLift coefficientThe lift coefficient is a dimensionless coefficient that relates the lift generated by a lifting body, the dynamic pressure of the fluid flow around the body, and a reference area associated with the body... is the 3D lift coefficientLift coefficientThe lift coefficient is a dimensionless coefficient that relates the lift generated by a lifting body, the dynamic pressure of the fluid flow around the body, and a reference area associated with the body...
is the 3D lift coefficientLift coefficientThe lift coefficient is a dimensionless coefficient that relates the lift generated by a lifting body, the dynamic pressure of the fluid flow around the body, and a reference area associated with the body...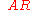 is the aspect ratioAspect ratio (wing)In aerodynamics, the aspect ratio of a wing is essentially the ratio of its length to its breadth . A high aspect ratio indicates long, narrow wings, whereas a low aspect ratio indicates short, stubby wings....
is the aspect ratioAspect ratio (wing)In aerodynamics, the aspect ratio of a wing is essentially the ratio of its length to its breadth . A high aspect ratio indicates long, narrow wings, whereas a low aspect ratio indicates short, stubby wings.... is an angle of attackAngle of attackAngle of attack is a term used in fluid dynamics to describe the angle between a reference line on a lifting body and the vector representing the relative motion between the lifting body and the fluid through which it is moving...
is an angle of attackAngle of attackAngle of attack is a term used in fluid dynamics to describe the angle between a reference line on a lifting body and the vector representing the relative motion between the lifting body and the fluid through which it is moving...
in radians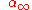 is the freestream angle of attack
is the freestream angle of attack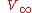 is the freestream velocity
is the freestream velocity is the drag coefficient for induced drag
is the drag coefficient for induced drag is the planform efficiency factor
is the planform efficiency factor
The following are functions of the wings span-wise station (i.e. can vary along the wing)
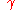 is the 2D circulation at a section
is the 2D circulation at a section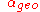 is the change in angle of attack due to geometric twist of the wing
is the change in angle of attack due to geometric twist of the wing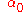 is zero lift angle of attack of that section
is zero lift angle of attack of that section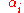 is change in angle of attack due to downwash
is change in angle of attack due to downwash is the chord length of the local section
is the chord length of the local section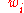 is the downwash
is the downwash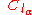 is the 2D lift coefficient slope (see Thin Airfoil Theory)
is the 2D lift coefficient slope (see Thin Airfoil Theory)
To derive the model we start with the assumption that the circulation of the wing varies as a function of the spanwise locations. The function assumed is a Fourier function. Firstly, the coordinate for the spanwise location
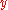 is transformed by
is transformed by 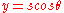 , where y is spanwise location, and s is the semi-span of the wing.
, where y is spanwise location, and s is the semi-span of the wing.and so the circulation is assumed to be:
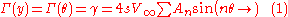
Since the circulation of a section is related the
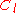 by the equation:
by the equation: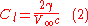
but since the coefficient of lift is a function of angle of attack:
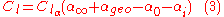
hence the vortex strength at any particular spanwise station can be given by the equations:
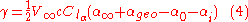
This one equation has two unknowns: the value for
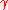 and the value for
and the value for 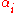 . However, the downwash is purely a function of the circulation only. So we can determine the value
. However, the downwash is purely a function of the circulation only. So we can determine the value 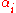 in terms of
in terms of 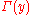 , bring this term across to the left hand side of the equation and solve. The downwash at any given station is a function of the entire shed vortex system. This is determined by integrating the influence of each differential shed vortex over the span of the wing.
, bring this term across to the left hand side of the equation and solve. The downwash at any given station is a function of the entire shed vortex system. This is determined by integrating the influence of each differential shed vortex over the span of the wing.Differential element of circulation:
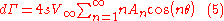
Differential downwash due to the differential element of circulation (acts like half an infinite vortex line):
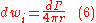
The integral equation over the span of the wing to determine the downwash at a particular location is:
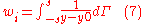
After appropriate substitutions and integrations we get:
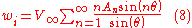
And so the change in angle attack is determined by (assuming small angles):
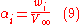
By substituting equations 8 and 9 into RHS of equation 4 and equation 1 into the LHS of equation 4, we then get:
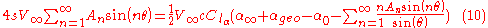
After rearranging, we get the series of simultaneous equations:


By taking a finite number of terms, equation 11 can be expressed in matrix form and solved for coefficients A. Note the left-hand side of the equation represents each element in the matrix, and the terms on the RHS of equation 11 represent the RHS of the matrix form. Each row in the matrix form represents a different span-wise station, and each column represents a different value for n.
Appropriate choices for
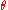 are as a linear variation between
are as a linear variation between  . Note that this range does not include the values for
. Note that this range does not include the values for  , as this will lead to a singular matrix, which can't be solved.
, as this will lead to a singular matrix, which can't be solved.Lift and Drag from coefficients
The lift can be determined by integrating the circulation terms: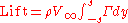
which can be reduced to:

The induced drag can be determined from
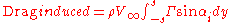
or
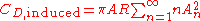
Symmetric Wing
For a symmetric wing, the even terms of the series coefficients are identically equal to 0, and so can be dropped.Rolling wings
When the aircraft is rolling, and additional term may be added that will add the wing station distance multiplied by the rate of roll to give additional angle of attack change. Equation 3 will then become

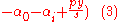
where
 is the rate of roll in rad/sec,
is the rate of roll in rad/sec,
Note that y can be negative. This addition will introduce non-zero even coefficients in the equation which will have to accounted for.
Control Deflection
The effect of ailerons can be accounted for simply changing term in Equation 3. For non-symmetric controls such as ailerons the
term in Equation 3. For non-symmetric controls such as ailerons the  term will change on each side of the wing.
term will change on each side of the wing.Elliptical Wings
For an elliptical wing with no twist, the chord length is given as a function of span location as:
Useful approximations
A useful approximation is that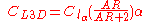
where
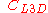 is the 3D lift coefficientLift coefficientThe lift coefficient is a dimensionless coefficient that relates the lift generated by a lifting body, the dynamic pressure of the fluid flow around the body, and a reference area associated with the body...
is the 3D lift coefficientLift coefficientThe lift coefficient is a dimensionless coefficient that relates the lift generated by a lifting body, the dynamic pressure of the fluid flow around the body, and a reference area associated with the body...
for elliptical circulation distribution,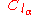 is the 2D lift coefficient slope (see Thin Airfoil Theory),
is the 2D lift coefficient slope (see Thin Airfoil Theory),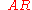 is the aspect ratioAspect ratio (wing)In aerodynamics, the aspect ratio of a wing is essentially the ratio of its length to its breadth . A high aspect ratio indicates long, narrow wings, whereas a low aspect ratio indicates short, stubby wings....
is the aspect ratioAspect ratio (wing)In aerodynamics, the aspect ratio of a wing is essentially the ratio of its length to its breadth . A high aspect ratio indicates long, narrow wings, whereas a low aspect ratio indicates short, stubby wings....
, and is the angle of attackAngle of attackAngle of attack is a term used in fluid dynamics to describe the angle between a reference line on a lifting body and the vector representing the relative motion between the lifting body and the fluid through which it is moving...
is the angle of attackAngle of attackAngle of attack is a term used in fluid dynamics to describe the angle between a reference line on a lifting body and the vector representing the relative motion between the lifting body and the fluid through which it is moving...
in radians.
The theoretical value for
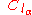 is 2
is 2 . Note that this equation becomes the thin airfoil equation if AR goes to infinity.
. Note that this equation becomes the thin airfoil equation if AR goes to infinity. Lifting-line theory also states an equation for induced drag:.

where
 is the drag coefficient for induced drag,
is the drag coefficient for induced drag, is the lift coefficientLift coefficientThe lift coefficient is a dimensionless coefficient that relates the lift generated by a lifting body, the dynamic pressure of the fluid flow around the body, and a reference area associated with the body...
is the lift coefficientLift coefficientThe lift coefficient is a dimensionless coefficient that relates the lift generated by a lifting body, the dynamic pressure of the fluid flow around the body, and a reference area associated with the body...
, and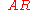 is the aspect ratioAspect ratio (wing)In aerodynamics, the aspect ratio of a wing is essentially the ratio of its length to its breadth . A high aspect ratio indicates long, narrow wings, whereas a low aspect ratio indicates short, stubby wings....
is the aspect ratioAspect ratio (wing)In aerodynamics, the aspect ratio of a wing is essentially the ratio of its length to its breadth . A high aspect ratio indicates long, narrow wings, whereas a low aspect ratio indicates short, stubby wings....
. is the planform efficiency factor (equals 1 for elliptical circulation distribution).
is the planform efficiency factor (equals 1 for elliptical circulation distribution).
Limitations of the theory
The lifting line theory does not take into account the following:- Compressible flow
- Viscous flow
- Swept wings
- Low aspect ratio wings
- Unsteady flows

