
Lambert summation
Encyclopedia
In mathematical analysis
, Lambert summation is a summability method for a class of divergent series
.
 is Lambert summable to A, written
is Lambert summable to A, written 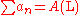 , if
, if
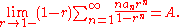
If a series is convergent to A then it is Lambert summable to A (an Abelian theorem).
Mathematical analysis
Mathematical analysis, which mathematicians refer to simply as analysis, has its beginnings in the rigorous formulation of infinitesimal calculus. It is a branch of pure mathematics that includes the theories of differentiation, integration and measure, limits, infinite series, and analytic functions...
, Lambert summation is a summability method for a class of divergent series
Divergent series
In mathematics, a divergent series is an infinite series that is not convergent, meaning that the infinite sequence of the partial sums of the series does not have a limit....
.
Definition
A series is Lambert summable to A, written
is Lambert summable to A, written 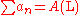 , if
, if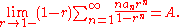
If a series is convergent to A then it is Lambert summable to A (an Abelian theorem).
Examples
-
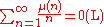 , where μ is the Möbius functionMöbius functionThe classical Möbius function μ is an important multiplicative function in number theory and combinatorics. The German mathematician August Ferdinand Möbius introduced it in 1832...
, where μ is the Möbius functionMöbius functionThe classical Möbius function μ is an important multiplicative function in number theory and combinatorics. The German mathematician August Ferdinand Möbius introduced it in 1832...
. Hence if this series converges at all, it converges to zero.

