Isogeny
Encyclopedia
In mathematics, an isogeny is a morphism
of varieties between two abelian varieties (e.g. elliptic curves) that is surjective and has a finite kernel.
Every isogeny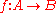
is automatically a group homomorphism
between the groups of k-valued points of
and 
, for any field k over which 
is defined.
Let
and 
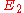be elliptic curves over a field k. An isogeny between 
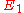and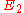 
is a surjective morphism 
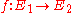of varieties that preserves basepoints (i.e. 
maps the infinite point on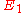 
to that on 
).
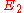Two elliptic curves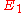
and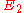 
are called isogenous if there is an isogeny 
. This is an equivalence relation, symmetry being due to the existence of the dual isogeny. As above, every isogeny induces homomorphisms of the groups of the k-valued points of the elliptic curves.
Morphism
In mathematics, a morphism is an abstraction derived from structure-preserving mappings between two mathematical structures. The notion of morphism recurs in much of contemporary mathematics...
of varieties between two abelian varieties (e.g. elliptic curves) that is surjective and has a finite kernel.
Every isogeny
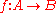is automatically a group homomorphismGroup homomorphism
In mathematics, given two groups and , a group homomorphism from to is a function h : G → H such that for all u and v in G it holds that h = h \cdot h...
between the groups of k-valued points of
and , for any field k over which is defined.Etymology
From the Greek (iso-) and Latin (genus), the term isogeny means "equal origins", a reference to the geometrical fact that an isogeny sends the point at infinity (the origin) of the source elliptic curve to the point at infinity of the target elliptic curve.Case of elliptic curves
For elliptic curves, this notion can also be formulated as follows:Let
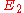and be elliptic curves over a field k. An isogeny between 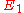and 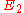is a surjective morphism 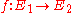of varieties that preserves basepoints (i.e. maps the infinite point on 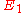to that on 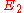).Two elliptic curves
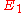and 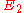are called isogenous if there is an isogeny . This is an equivalence relation, symmetry being due to the existence of the dual isogeny. As above, every isogeny induces homomorphisms of the groups of the k-valued points of the elliptic curves.