Factorization system
Encyclopedia
In mathematics
, it can be shown that every function
can be written as the composite of a surjective function followed by an injective function. Factorization systems are a generalization of this situation in category theory
.
and 
are said to be orthogonal, denoted 
, if for every pair of morphisms 
and 
such that 
there is a unique morphism 
such that the diagram
commutes. This notion can be extended to define the orthogonals of sets of morphisms by
and 
Since in a factorization system
contains all the isomorphisms, the condition (3) of the definition is equivalent to 
and 
of classes of morphisms of C is a factorization system if and only if it satisfies the following conditions:
and 
are two morphisms in a category C. Then 
has the left lifting property with respect to 
(resp. 
has the right lifting property with respect to 
) when for every pair of morphisms 
and 
such that 
there is a (not necessarily unique!) morphism 
such that the diagram
commutes.
A weak factorization system (E, M) for a category C consists of two classes of morphisms E and M of C such that :
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, it can be shown that every function
Function (mathematics)
In mathematics, a function associates one quantity, the argument of the function, also known as the input, with another quantity, the value of the function, also known as the output. A function assigns exactly one output to each input. The argument and the value may be real numbers, but they can...
can be written as the composite of a surjective function followed by an injective function. Factorization systems are a generalization of this situation in category theory
Category theory
Category theory is an area of study in mathematics that examines in an abstract way the properties of particular mathematical concepts, by formalising them as collections of objects and arrows , where these collections satisfy certain basic conditions...
.
Definition
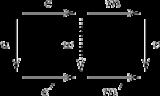
A factorization system (E, M) for a category C consists of two classes of morphisms E and M of C such that:- E and M both contain all isomorphisms of C and are closed under composition.
- Every morphism f of C can be factored as
for some morphisms 
and 
. - The factorization is functorial: if
and 
are two morphisms such that 
for some morphisms 
and 
, then there exists a unique morphism 
making the following diagram commute:
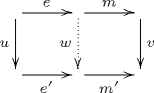
Orthogonality
Two morphisms and are said to be orthogonal, denoted , if for every pair of morphisms and such that there is a unique morphism such that the diagramcommutes. This notion can be extended to define the orthogonals of sets of morphisms by
and Since in a factorization system
contains all the isomorphisms, the condition (3) of the definition is equivalent to and Equivalent definition
The pair of classes of morphisms of C is a factorization system if and only if it satisfies the following conditions:- Every morphism f of C can be factored as
with 
and 
and 
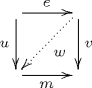
Weak factorization systems
Suppose and are two morphisms in a category C. Then has the left lifting property with respect to (resp. has the right lifting property with respect to ) when for every pair of morphisms and such that there is a (not necessarily unique!) morphism such that the diagramcommutes.
A weak factorization system (E, M) for a category C consists of two classes of morphisms E and M of C such that :
- The class E is exactly the class of morphisms having the left lifting property wrt the morphisms of M.
- The class M is exactly the class of morphisms having the right lifting property wrt the morphisms of E.
- Every morphism f of C can be factored as
for some morphisms 
and 
.