Dots and Boxes
Encyclopedia
Dots and Boxes is a pencil and paper game for two players (or sometimes, more than two) first published in 1889 by Édouard Lucas
.
Starting with an empty grid of dots, players take turns, adding a single horizontal or vertical line between two unjoined adjacent dots. A player who completes the fourth side of a 1×1 box earns one point and takes another turn. (The points are typically recorded by placing in the box an identifying mark of the player, such as an initial). The game ends when no more lines can be placed. The winner of the game is the player with the most points.
The board may be of any size. When short on time, 2×2 boxes (created by a square of 9 dots) is good for beginners, and 6×6 is good for experts. In games with an even number of boxes, it is conventional that if the game is tied then the win should be awarded to the second player (this offsets the advantage of going first).
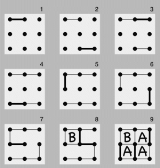
The diagram on the right shows a game being played on the 2×2 board. The second player (B) plays the mirror image of the first player's move, hoping to divide the board into two pieces and tie the game. The first player (A) makes a sacrifice at move 7; B accepts the sacrifice, getting one box. However, B must now add another line, and connects the center dot to the center-right dot, causing the remaining boxes to be joined together in a chain as shown at the end of move 8. With A's next move, A gets them all, winning 3–1.
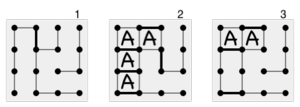At the start of a game, play is more or less random, the only strategy is to avoid adding the third side to any box. This continues until all the remaining (potential) boxes are joined together into chains – groups of one or more adjacent boxes in which any move gives all the boxes in the chain to the opponent. A novice player faced with a situation like position 1 in the diagram on the left, in which some boxes can be captured, takes all the boxes in the chain, resulting in position 2. But with their last move, they have to open the next (and larger) chain, and the novice loses the game,
An experienced player faced with position 1 instead plays the double-cross strategy, taking all but 2 of the boxes in the chain, leaving position 3. This leaves the last two boxes in the chain for their opponent, but then the opponent has to open the next chain. By moving to position 3, player A wins.
The double-cross strategy applies however many long chains there are. Take all but two of the boxes in each chain, but take all the boxes in the last chain. If the chains are long enough then the player will certainly win. Therefore, when played by experts, Dots and Boxes becomes a battle for control: An expert player tries to force their opponent to start the first long chain. Against a player who doesn't understand the concept of a sacrifice, the expert simply has to make the correct number of sacrifices to encourage the opponent to hand him the first chain long enough to ensure a win. If the other player also knows to offer sacrifices, the expert also has to manipulate the number of available sacrifices through earlier play.
There is never any reason not to accept a sacrifice, as if it is refused, the player who offered it can always take it without penalty. Thus, the impact of refusing a sacrifice need not be considered in your strategy.
Experienced players can avoid the chaining phenomenon by making early moves to split the board. A board split into 4x4 squares is ideal. Dividing limits the size of chains- in the case of 4x4 squares, the longest possible chain is four, filling the larger square. A board with an even number of spaces will end in a draw (as the number of 4x4 squares will be equal for each player); an odd numbered board will lead to the winner winning by one square (the 4x4 squares and 2x1 half-squares will fall evenly, with one box not incorporated into the pattern falling to the winner).
A common alternate ruleset is to require all available boxes be claimed on your turn. This eliminates the double cross strategy, forcing even the experienced player to take all the boxes, and give his opponent the win.
In combinatorial game theory
dots and boxes is very close to being an impartial game
and many positions can be analyzed using Sprague–Grundy theory
.
when it is played in a Chakana
or Inca Cross grid, which adds more complications to the game.
Dots-and-boxes has a dual
form called "strings-and-coins". This game is played on a network of coins (vertices) joined by strings (edges). Players take turns to cut a string. When a cut leaves a coin with no strings, the player pockets the coin and takes another turn. The winner is the player who pockets the most coins. Strings-and-coins can be played on an arbitrary graph
.
A variant played in Poland allows a player to claim a region of several squares as soon as its boundary is completed.
Edouard Lucas
François Édouard Anatole Lucas was a French mathematician. Lucas is known for his study of the Fibonacci sequence. The related Lucas sequences and Lucas numbers are named after him.-Biography:...
.
Starting with an empty grid of dots, players take turns, adding a single horizontal or vertical line between two unjoined adjacent dots. A player who completes the fourth side of a 1×1 box earns one point and takes another turn. (The points are typically recorded by placing in the box an identifying mark of the player, such as an initial). The game ends when no more lines can be placed. The winner of the game is the player with the most points.
The board may be of any size. When short on time, 2×2 boxes (created by a square of 9 dots) is good for beginners, and 6×6 is good for experts. In games with an even number of boxes, it is conventional that if the game is tied then the win should be awarded to the second player (this offsets the advantage of going first).
The diagram on the right shows a game being played on the 2×2 board. The second player (B) plays the mirror image of the first player's move, hoping to divide the board into two pieces and tie the game. The first player (A) makes a sacrifice at move 7; B accepts the sacrifice, getting one box. However, B must now add another line, and connects the center dot to the center-right dot, causing the remaining boxes to be joined together in a chain as shown at the end of move 8. With A's next move, A gets them all, winning 3–1.
Strategy
An experienced player faced with position 1 instead plays the double-cross strategy, taking all but 2 of the boxes in the chain, leaving position 3. This leaves the last two boxes in the chain for their opponent, but then the opponent has to open the next chain. By moving to position 3, player A wins.
The double-cross strategy applies however many long chains there are. Take all but two of the boxes in each chain, but take all the boxes in the last chain. If the chains are long enough then the player will certainly win. Therefore, when played by experts, Dots and Boxes becomes a battle for control: An expert player tries to force their opponent to start the first long chain. Against a player who doesn't understand the concept of a sacrifice, the expert simply has to make the correct number of sacrifices to encourage the opponent to hand him the first chain long enough to ensure a win. If the other player also knows to offer sacrifices, the expert also has to manipulate the number of available sacrifices through earlier play.
There is never any reason not to accept a sacrifice, as if it is refused, the player who offered it can always take it without penalty. Thus, the impact of refusing a sacrifice need not be considered in your strategy.
Experienced players can avoid the chaining phenomenon by making early moves to split the board. A board split into 4x4 squares is ideal. Dividing limits the size of chains- in the case of 4x4 squares, the longest possible chain is four, filling the larger square. A board with an even number of spaces will end in a draw (as the number of 4x4 squares will be equal for each player); an odd numbered board will lead to the winner winning by one square (the 4x4 squares and 2x1 half-squares will fall evenly, with one box not incorporated into the pattern falling to the winner).
A common alternate ruleset is to require all available boxes be claimed on your turn. This eliminates the double cross strategy, forcing even the experienced player to take all the boxes, and give his opponent the win.
In combinatorial game theory
Combinatorial game theory
Combinatorial game theory is a branch of applied mathematics and theoretical computer science that studies sequential games with perfect information, that is, two-player games which have a position in which the players take turns changing in defined ways or moves to achieve a defined winning...
dots and boxes is very close to being an impartial game
Impartial game
In combinatorial game theory, an impartial game is a game in which the allowable moves depend only on the position and not on which of the two players is currently moving, and where the payoffs are symmetric...
and many positions can be analyzed using Sprague–Grundy theory
Sprague–Grundy theorem
In combinatorial game theory, the Sprague–Grundy theorem states that every impartial game under the normal play convention is equivalent to a nimber. The Grundy value or nim-value of an impartial game is then defined as the unique nimber that the game is equivalent to...
.
Unusual grids
Dots and boxes need not be played on a rectangular grid. It can be played on a triangular grid or a hexagonal grid. There is also a variant in BoliviaBolivia
Bolivia officially known as Plurinational State of Bolivia , is a landlocked country in central South America. It is the poorest country in South America...
when it is played in a Chakana
Chakana
The Chakana symbolizes for Inca mythology what is known in other mythologies as the World Tree, Tree of Life and so on. The stepped cross is made up of an equal-armed cross indicating the cardinal points of the compass and a superimposed square. The square represents the other two levels of...
or Inca Cross grid, which adds more complications to the game.
Dots-and-boxes has a dual
Dual graph
In mathematics, the dual graph of a given planar graph G is a graph which has a vertex for each plane region of G, and an edge for each edge in G joining two neighboring regions, for a certain embedding of G. The term "dual" is used because this property is symmetric, meaning that if H is a dual...
form called "strings-and-coins". This game is played on a network of coins (vertices) joined by strings (edges). Players take turns to cut a string. When a cut leaves a coin with no strings, the player pockets the coin and takes another turn. The winner is the player who pockets the most coins. Strings-and-coins can be played on an arbitrary graph
Graph (mathematics)
In mathematics, a graph is an abstract representation of a set of objects where some pairs of the objects are connected by links. The interconnected objects are represented by mathematical abstractions called vertices, and the links that connect some pairs of vertices are called edges...
.
A variant played in Poland allows a player to claim a region of several squares as soon as its boundary is completed.