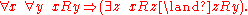Dense relation
Encyclopedia
In mathematics
, a binary relation
R is said to be dense if, for all R-related x and y, there is a z such that x and z and also z and y are R-related.
Formally:
For example, a strict partial order < is a dense order
iff
< is a dense relation.
Every reflexive relation
is dense.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, a binary relation
Binary relation
In mathematics, a binary relation on a set A is a collection of ordered pairs of elements of A. In other words, it is a subset of the Cartesian product A2 = . More generally, a binary relation between two sets A and B is a subset of...
R is said to be dense if, for all R-related x and y, there is a z such that x and z and also z and y are R-related.
Formally:
For example, a strict partial order < is a dense order
Dense order
In mathematics, a partial order ≤ on a set X is said to be dense if, for all x and y in X for which x In mathematics, a partial order ≤ on a set X is said to be dense if, for all x and y in X for which x...
iff
IFF
IFF, Iff or iff may refer to:Technology/Science:* Identification friend or foe, an electronic radio-based identification system using transponders...
< is a dense relation.
Every reflexive relation
Reflexive relation
In mathematics, a reflexive relation is a binary relation on a set for which every element is related to itself, i.e., a relation ~ on S where x~x holds true for every x in S. For example, ~ could be "is equal to".-Related terms:...
is dense.