Comprehensive School Mathematics Program
Encyclopedia
Comprehensive School Mathematics Program (CSMP) stands for both the name of a curriculum and the name of the project that was responsible for developing curriculum materials.
Two major curricula were developed under CSMP project, Comprehensive School Mathematics Program(CSMP), a K-6 mathematics program for regular classroom instruction, and the Elements of Mathematics (EM) program, a grades 7-12 mathematics program for gifted students. EM treats traditional topics rigorously and in depth and was the only curriculum that strictly adhere to the Goals for School Mathematics: The Report of the Cambridge Conference on School Mathematics (1963). As a result, it includes much of the content generally required for an undergraduate mathematics major. These two curricula are unrelated to one another but certain members of the CSMP staff contributed to the development of both projects. The Elements of Mathematics is widely used at the IMACS institute listed below. What follows is a description of the K-6 program that was designed for a general heterogeneous audience.
The CSMP Project was established in 1966, under the direction of Burt Kaufman, who remained director until 1979 when Clare Heidema became director until 2003. It was originally affiliated with Southern Illinois University in Carbondale, Illinois. After a year of planning, CSMP was incorporated into the Central Midwest Regional Educational Laboratory (later CEMREL, Inc.), one of the national educational laboratories funded at that time by the U.S. Office of Education. (see Final Evaluation Report by Martin Herbert referenced below for more detail) . In 1984, the project moved to Mid-continental Research for Learning (McREL) Institute's Comprehensive School Reform program, who supported the program until 2003. Clare Heidema remained director to its conclusion. In 1984, it was implemented in 150 school districts in 42 states and about 55,000 students.
with a bag of magic peanuts — some representing positive integers, some negative.
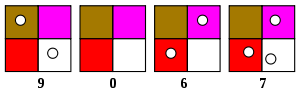One device used throughout the program was a mini-computer. This was a 2 by 2 grid of squares, the squares represented the numbers 1, 2, 4, and 8. Checkers could be placed on the grid to represent different numbers in a similar fashion to the way the binary numeral system
is used to represent numbers in a computer
.
The mini-computer is laid out as follows: a white square in the lower right corner with a value of 1, a red square in the lower left with a value of 2, a purple square in the upper right with a value of 4, and a brown square in the upper left with a value of 8. Each mini-computer is designed to represent a single decimal digit, and multiple mini-computers can be used together to represent multiple-digit numbers. Each successive board's values are increased by a power of ten. For example, a second mini-computer's squares will represent 10, 20, 40, and 80; a third, 100, 200, 400, and 800, and so on.
Students are instructed to represent values on the mini-computers by adding checkers to the proper squares. To do this only requires a memorization of representations for the digits zero through nine, although non-standard representations are possible since squares can hold more than one checker. Each checker is worth the value of the square it is in, and the sum of the checkers on the board(s) determine the overall value represented. Most checkers used by students are a solid color- any color is fine. The only exception is checkers marked with a caret
(^), which are negative.
An example of representing a number: 9067 requires four boards. The leftmost board has two checkers in the 8 and 1 squares (8000 + 1000). The second board has none, as the value has zero hundreds. The third board has checkers in the 4 and 2 squares (40 + 20), and the rightmost board has checkers in the 4, 2, and 1 squares (4 + 2 + 1). Together, these 7 values (8000 + 1000 + 40 + 20 + 4 + 2 + 1) total up to 9067.
This would be considered a standard way to represent the number as it involves the fewest checkers possible without involving negatives. It would be simpler to replace the last board with a positive checker in the 8 and a negative checker in the 1, but this is not taught as the standard.
Arithmetic can be performed on the mini-computer by combining two numbers' representations into a single board and performing simplification techniques. One such technique is to replace checkers from the 8 and 2 squares of one board with a checker on the 1 square of the adjacent board to the left. Another technique is to replace a pair of checkers in the same square with one checker in the next higher square, such as two 4's with an 8.
Two major curricula were developed under CSMP project, Comprehensive School Mathematics Program(CSMP), a K-6 mathematics program for regular classroom instruction, and the Elements of Mathematics (EM) program, a grades 7-12 mathematics program for gifted students. EM treats traditional topics rigorously and in depth and was the only curriculum that strictly adhere to the Goals for School Mathematics: The Report of the Cambridge Conference on School Mathematics (1963). As a result, it includes much of the content generally required for an undergraduate mathematics major. These two curricula are unrelated to one another but certain members of the CSMP staff contributed to the development of both projects. The Elements of Mathematics is widely used at the IMACS institute listed below. What follows is a description of the K-6 program that was designed for a general heterogeneous audience.
The CSMP Project was established in 1966, under the direction of Burt Kaufman, who remained director until 1979 when Clare Heidema became director until 2003. It was originally affiliated with Southern Illinois University in Carbondale, Illinois. After a year of planning, CSMP was incorporated into the Central Midwest Regional Educational Laboratory (later CEMREL, Inc.), one of the national educational laboratories funded at that time by the U.S. Office of Education. (see Final Evaluation Report by Martin Herbert referenced below for more detail) . In 1984, the project moved to Mid-continental Research for Learning (McREL) Institute's Comprehensive School Reform program, who supported the program until 2003. Clare Heidema remained director to its conclusion. In 1984, it was implemented in 150 school districts in 42 states and about 55,000 students.
Overview
The most influential figure on this project was Frederque’ Papy. This project employs four non-verbal languages for the purpose of posing problems and representing mathematical concepts. They are: the Papy Minicomputer(mental computation), Arrows(relations), Strings(classification) and Calculators(patterns). It was designed to teach mathematics as a problem solving activity rather than just teaching arithmetic skills. The program was highly structured using the spiral scheme of program development. It introduced many basic concepts such as fractions earlier than normal but was criticized for lack of emphasis given to calculation. New content in probability and geometry was introduced. There was a range of supporting material including story books with mathematical problems. Lessons were often posed in a story. One character in these books was Eli the Elephant, a pachydermElephant
Elephants are large land mammals in two extant genera of the family Elephantidae: Elephas and Loxodonta, with the third genus Mammuthus extinct...
with a bag of magic peanuts — some representing positive integers, some negative.
The Mini-Computer
Binary numeral system
The binary numeral system, or base-2 number system, represents numeric values using two symbols, 0 and 1. More specifically, the usual base-2 system is a positional notation with a radix of 2...
is used to represent numbers in a computer
Computer
A computer is a programmable machine designed to sequentially and automatically carry out a sequence of arithmetic or logical operations. The particular sequence of operations can be changed readily, allowing the computer to solve more than one kind of problem...
.
The mini-computer is laid out as follows: a white square in the lower right corner with a value of 1, a red square in the lower left with a value of 2, a purple square in the upper right with a value of 4, and a brown square in the upper left with a value of 8. Each mini-computer is designed to represent a single decimal digit, and multiple mini-computers can be used together to represent multiple-digit numbers. Each successive board's values are increased by a power of ten. For example, a second mini-computer's squares will represent 10, 20, 40, and 80; a third, 100, 200, 400, and 800, and so on.
Students are instructed to represent values on the mini-computers by adding checkers to the proper squares. To do this only requires a memorization of representations for the digits zero through nine, although non-standard representations are possible since squares can hold more than one checker. Each checker is worth the value of the square it is in, and the sum of the checkers on the board(s) determine the overall value represented. Most checkers used by students are a solid color- any color is fine. The only exception is checkers marked with a caret
Caret
Caret usually refers to the spacing symbol ^ in ASCII and other character sets. In Unicode, however, the corresponding character is , whereas the Unicode character named caret is actually a similar but lowered symbol: ....
(^), which are negative.
An example of representing a number: 9067 requires four boards. The leftmost board has two checkers in the 8 and 1 squares (8000 + 1000). The second board has none, as the value has zero hundreds. The third board has checkers in the 4 and 2 squares (40 + 20), and the rightmost board has checkers in the 4, 2, and 1 squares (4 + 2 + 1). Together, these 7 values (8000 + 1000 + 40 + 20 + 4 + 2 + 1) total up to 9067.
This would be considered a standard way to represent the number as it involves the fewest checkers possible without involving negatives. It would be simpler to replace the last board with a positive checker in the 8 and a negative checker in the 1, but this is not taught as the standard.
Arithmetic can be performed on the mini-computer by combining two numbers' representations into a single board and performing simplification techniques. One such technique is to replace checkers from the 8 and 2 squares of one board with a checker on the 1 square of the adjacent board to the left. Another technique is to replace a pair of checkers in the same square with one checker in the next higher square, such as two 4's with an 8.