
Complex analytic geometry
Encyclopedia
In mathematics
, complex analytic geometry sometimes
denotes the application of complex number
s to plane geometry
.
Rather than represent a point in the plane as a pair of Cartesian coordinates
, it can be represented as a single complex number, which can be written at will in either rectangular or polar form.
Complex analytical geometry deals with solving geometrical problems, especially those involving angles, by means of complex algebra
. Using Euler's formula
,
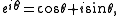
it is possible to represent any rotation
as a complex number.
Complex numbers can therefore be used to advantage to solve problems in elementary geometry
. This method is sometimes used in the advanced high-school mathematics, since it allows schoolchildren to write down angle relations in terms of simple formulas.
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, complex analytic geometry sometimes
denotes the application of complex number
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
s to plane geometry
Plane geometry
In mathematics, plane geometry may refer to:*Euclidean plane geometry, the geometry of plane figures,*geometry of a plane,or sometimes:*geometry of a projective plane, most commonly the real projective plane but possibly the complex projective plane, Fano plane or others;*geometry of the hyperbolic...
.
Rather than represent a point in the plane as a pair of Cartesian coordinates
Cartesian coordinate system
A Cartesian coordinate system specifies each point uniquely in a plane by a pair of numerical coordinates, which are the signed distances from the point to two fixed perpendicular directed lines, measured in the same unit of length...
, it can be represented as a single complex number, which can be written at will in either rectangular or polar form.
Complex analytical geometry deals with solving geometrical problems, especially those involving angles, by means of complex algebra
Complex number
A complex number is a number consisting of a real part and an imaginary part. Complex numbers extend the idea of the one-dimensional number line to the two-dimensional complex plane by using the number line for the real part and adding a vertical axis to plot the imaginary part...
. Using Euler's formula
Euler's formula
Euler's formula, named after Leonhard Euler, is a mathematical formula in complex analysis that establishes the deep relationship between the trigonometric functions and the complex exponential function...
,
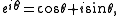
it is possible to represent any rotation
Rotation
A rotation is a circular movement of an object around a center of rotation. A three-dimensional object rotates always around an imaginary line called a rotation axis. If the axis is within the body, and passes through its center of mass the body is said to rotate upon itself, or spin. A rotation...
as a complex number.
Complex numbers can therefore be used to advantage to solve problems in elementary geometry
Geometry
Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....
. This method is sometimes used in the advanced high-school mathematics, since it allows schoolchildren to write down angle relations in terms of simple formulas.
External links
- Complex Numbers and Geometry (Important formulas and links to applications)

