
Cartan's lemma (potential theory)
Encyclopedia
In potential theory
, a branch of mathematics
, Cartan's lemma, named after Henri Cartan
, is a bound on the measure and complexity of the set on which a logarithmic Newtonian potential
is small. The following statement can be found in Levin's book.
Let μ be a finite positive Borel measure on the complex plane C with μ(C) = n. Let u(z) be the logarithmic potential of μ:
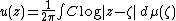
Given H ∈ (0, 1), there exist discs of radius ri such that
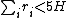
and
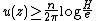
for all z outside the union of these discs.
Potential theory
In mathematics and mathematical physics, potential theory may be defined as the study of harmonic functions.- Definition and comments :The term "potential theory" was coined in 19th-century physics, when it was realized that the fundamental forces of nature could be modeled using potentials which...
, a branch of mathematics
Mathematics
Mathematics is the study of quantity, space, structure, and change. Mathematicians seek out patterns and formulate new conjectures. Mathematicians resolve the truth or falsity of conjectures by mathematical proofs, which are arguments sufficient to convince other mathematicians of their validity...
, Cartan's lemma, named after Henri Cartan
Henri Cartan
Henri Paul Cartan was a French mathematician with substantial contributions in algebraic topology. He was the son of the French mathematician Élie Cartan.-Life:...
, is a bound on the measure and complexity of the set on which a logarithmic Newtonian potential
Newtonian potential
In mathematics, the Newtonian potential or Newton potential is an operator in vector calculus that acts as the inverse to the negative Laplacian, on functions that are smooth and decay rapidly enough at infinity. As such, it is a fundamental object of study in potential theory...
is small. The following statement can be found in Levin's book.
Let μ be a finite positive Borel measure on the complex plane C with μ(C) = n. Let u(z) be the logarithmic potential of μ:
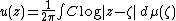
Given H ∈ (0, 1), there exist discs of radius ri such that
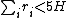
and
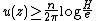
for all z outside the union of these discs.

