In geometry

Geometry

Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....

, Brianchon's theorem, named after Charles Julien Brianchon

Charles Julien Brianchon

Charles Julien Brianchon was a French mathematician and chemist. He entered into the École Polytechnique in 1804 at the age of eighteen, and studied under Monge, graduating first in his class in 1808, after which he took up a career as a lieutenant in Napoleon's artillery...

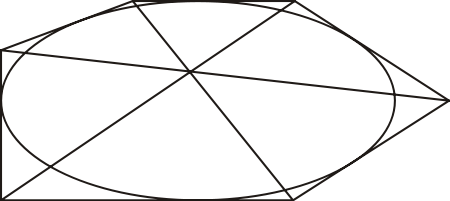
 (1783–1864), is as follows. Let ABCDEF be a hexagon formed by six tangent lines of a conic section

Conic section

In mathematics, a conic section is a curve obtained by intersecting a cone with a plane. In analytic geometry, a conic may be defined as a plane algebraic curve of degree 2...

. Then lines AD, BE, CF intersect at a single point

Point (geometry)

In geometry, topology and related branches of mathematics a spatial point is a primitive notion upon which other concepts may be defined. In geometry, points are zero-dimensional; i.e., they do not have volume, area, length, or any other higher-dimensional analogue. In branches of mathematics...

.

The polar reciprocal and projective dual of this theorem give Pascal's theorem

Pascal's theorem

In projective geometry, Pascal's theorem states that if an arbitrary hexagon is inscribed in any conic section, and pairs of opposite sides are extended until they meet, the three intersection points will lie on a straight line, the Pascal line of that configuration.- Related results :This theorem...

.

Brianchon's theorem is true in both the affine plane and the real projective plane

Real projective plane

In mathematics, the real projective plane is an example of a compact non-orientable two-dimensional manifold, that is, a one-sided surface. It cannot be embedded in our usual three-dimensional space without intersecting itself...

. However, its statement in the affine plane is in a sense less informative and more complicated than that in the projective plane

Projective plane

In mathematics, a projective plane is a geometric structure that extends the concept of a plane. In the ordinary Euclidean plane, two lines typically intersect in a single point, but there are some pairs of lines that do not intersect...

. Consider, for example, five tangent lines to a parabola

Parabola

In mathematics, the parabola is a conic section, the intersection of a right circular conical surface and a plane parallel to a generating straight line of that surface...

. These may be considered sides of a hexagon whose sixth side is the line at infinity

Line at infinity

In geometry and topology, the line at infinity is a line that is added to the real plane in order to give closure to, and remove the exceptional cases from, the incidence properties of the resulting projective plane. The line at infinity is also called the ideal line.-Geometric formulation:In...

, but there is no line at infinity in the affine plane (nor in the projective plane unless one chooses a line to play that role). A line from a vertex to the opposite vertex would then be a line parallel to one of the five tangent lines. Brianchon's theorem stated only for the affine plane would be uninformative about such a situation.

The projective dual of Brianchon's theorem has exceptions in the affine plane but not in the projective plane.

Brianchon's theorem can be proved by the idea of radical axis

Radical axis

The radical axis of two circles is the locus of points at which tangents drawn to both circles have the same length. For any point P on the radical axis, there is a unique circle centered on P that intersects both circles at right angles ; conversely, the center of any circle that cuts both...

or reciprocation.