Brahmagupta's theorem is a result in geometry

Geometry

Geometry arose as the field of knowledge dealing with spatial relationships. Geometry was one of the two fields of pre-modern mathematics, the other being the study of numbers ....

. It states that if a cyclic quadrilateral

Cyclic quadrilateral

In Euclidean geometry, a cyclic quadrilateral is a quadrilateral whose vertices all lie on a single circle. This circle is called the circumcircle or circumscribed circle, and the vertices are said to be concyclic. Other names for these quadrilaterals are chordal quadrilateral and inscribed...

 is orthodiagonal

Orthodiagonal quadrilateral

In Euclidean geometry, an orthodiagonal quadrilateral is a quadrilateral in which the diagonals cross at right angles. In other words, it is a four-sided figure in which the line segments between non-adjacent vertices are orthogonal to each other....

 (that is, has perpendicular

Perpendicular

In geometry, two lines or planes are considered perpendicular to each other if they form congruent adjacent angles . The term may be used as a noun or adjective...

 diagonals), then the perpendicular to a side from the point of intersection of the diagonals always bisects the opposite side. It is named after the Indian mathematician Brahmagupta

Brahmagupta

Brahmagupta was an Indian mathematician and astronomer who wrote many important works on mathematics and astronomy. His best known work is the Brāhmasphuṭasiddhānta , written in 628 in Bhinmal...

.

More specifically, let A, B, C and D be four points on a circle such that the lines AC and BD are perpendicular. Denote the intersection of AC and BD by M. Drop the perpendicular from M to the line BC, calling the intersection E. Let F be the intersection of the line EM and the edge AD. Then, the theorem states that F is the midpoint AD.

Proof

We need to prove that AF = FD. We will prove that both AF and FD are in fact equal to FM.

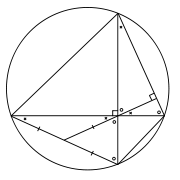
To prove that AF = FM, first note that the angles FAM and CBM are equal, because they are inscribed angle

Inscribed angle

In geometry, an inscribed angle is formed when two secant lines of a circle intersect on the circle....

s that intercept the same arc of the circle. Furthermore, the angles CBM and CME are both complementary

Complementary angles

In geometry, complementary angles are angles whose measures sum to 90°. If the two complementary angles are adjacent their non-shared sides form a right angle....

 to angle BCM (i.e., they add up to 90°), and are therefore equal. Finally, the angles CME and FMA are the same. Hence, AFM is an isosceles triangle, and thus the sides AF and FM are equal.

The proof that FD = FM goes similarly: the angles FDM, BCM, BME and DMF are all equal, so DFM is an isosceles triangle, so FD = FM. It follows that AF = FD, as the theorem claims.

External links

• Brahmagupta's Theorem at cut-the-knot
  Cut-the-knot
  Cut-the-knot is a free, advertisement-funded educational website maintained by Alexander Bogomolny and devoted to popular exposition of many topics in mathematics. The site has won more than 20 awards from scientific and educational publications, including a Scientific American Web Award in 2003,...