
Appell–Humbert theorem
Encyclopedia
In mathematics, the Appell–Humbert theorem describes the line bundle
s on a complex torus
or complex abelian variety
.
It was proved for 2-dimensional tori by and .

then
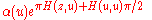
is a 1-cocycle
on U defining a line bundle on T.
The Appell–Humbert theorem says that every line bundle on T can be constructed like this for a unique choice of H and α satisfying the conditions above.
Line bundle
In mathematics, a line bundle expresses the concept of a line that varies from point to point of a space. For example a curve in the plane having a tangent line at each point determines a varying line: the tangent bundle is a way of organising these...
s on a complex torus
Complex torus
In mathematics, a complex torus is a particular kind of complex manifold M whose underlying smooth manifold is a torus in the usual sense...
or complex abelian variety
Abelian variety
In mathematics, particularly in algebraic geometry, complex analysis and number theory, an abelian variety is a projective algebraic variety that is also an algebraic group, i.e., has a group law that can be defined by regular functions...
.
It was proved for 2-dimensional tori by and .
Statement
Suppose that T is a complex torus given by V/U where U is a lattice in a complex vector space V. If H is a Hermitian form on V whose imaginary part is integral on U×U, and α is a map from U to the unit circle such that
then
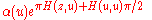
is a 1-cocycle
Cocycle
A cocycle refers to any one of the following:*A closed cochain in algebraic topology is called a cocycle.*A particular type of map in an autonomous dynamical system; see Oseledec theorem....
on U defining a line bundle on T.
The Appell–Humbert theorem says that every line bundle on T can be constructed like this for a unique choice of H and α satisfying the conditions above.

