
Adiabatic accessibility
Encyclopedia
Adiabatic accessibility denotes a certain relation between two equilibrium states of a thermodynamic system
(or of different such systems). The concept was coined by Constantin Carathéodory
in 1909 ("adiabatische Erreichbarkeit") and taken up 90 years later by Elliott Lieb
and J. Yngvason
in their axiomatic approach to the foundations of thermodynamics . It was also used by R. Giles in his 1964 monograph.
The original definition of Carathéodory was limited to reversible, quasistatic process, described by a curve in the manifold of equilibrium states of the system under consideration. He called such a state change adiabatic if the infinitesimal 'heat' differential form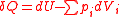
vanishes along the curve. In other words, at no time in the process does heat enter or leave the system. Carathéodory's formulation of the Second Law of Thermodynamics
then takes the form: "In the neighbourhood of any initial state, there are states which cannot be approached arbitrarily close through adiabatic changes of state." From this principle he derived the existence of entropy
as a state function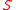
whose differential is proportional to the heat differential form
is proportional to the heat differential form 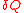 , so it remains constant under adiabatic state changes (in Carathéodory's sense). The increase of entropy during irreversible
, so it remains constant under adiabatic state changes (in Carathéodory's sense). The increase of entropy during irreversible
processes is not obvious in this formulation, without further assumptions.
The definition employed by Lieb and Yngvason is rather different since the state changes considered can be the result of arbitrarily complicated, possibly violent, irreversible processes and there is no mention of 'heat' or differential forms. It goes as follows: A state is adiabatically accessible from a state
is adiabatically accessible from a state  , in symbols
, in symbols  , if it is possible to transform
, if it is possible to transform  into
into  in such a way that the only net effect of the process on the surroundings is that a weight has been raised or lowered (or a spring is stretched/compressed, or a flywheel is set in motion).
in such a way that the only net effect of the process on the surroundings is that a weight has been raised or lowered (or a spring is stretched/compressed, or a flywheel is set in motion).
A definition of thermodynamic entropy can be based entirely on certain properties of the relation of adiabatic accessibility that are taken as axioms in the Lieb-Yngvason approach. The entropy has the property that
of adiabatic accessibility that are taken as axioms in the Lieb-Yngvason approach. The entropy has the property that 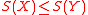 if and only if
if and only if  , in accord with the Second Law.
, in accord with the Second Law.
Thermodynamic system
A thermodynamic system is a precisely defined macroscopic region of the universe, often called a physical system, that is studied using the principles of thermodynamics....
(or of different such systems). The concept was coined by Constantin Carathéodory
Constantin Carathéodory
Constantin Carathéodory was a Greek mathematician. He made significant contributions to the theory of functions of a real variable, the calculus of variations, and measure theory...
in 1909 ("adiabatische Erreichbarkeit") and taken up 90 years later by Elliott Lieb
Elliott H. Lieb
Elliott H. Lieb is an eminent American mathematical physicist and professor of mathematics and physics at Princeton University who specializes in statistical mechanics, condensed matter theory, and functional analysis....
and J. Yngvason
Jakob Yngvason
Jakob Yngvason is an Icelandic/Austrian physicist. He is professor of mathematical physics at the University of Vienna and Scientific Director of the Erwin Schrödinger International Institute for Mathematical Physics in Vienna...
in their axiomatic approach to the foundations of thermodynamics . It was also used by R. Giles in his 1964 monograph.
The original definition of Carathéodory was limited to reversible, quasistatic process, described by a curve in the manifold of equilibrium states of the system under consideration. He called such a state change adiabatic if the infinitesimal 'heat' differential form
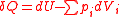
vanishes along the curve. In other words, at no time in the process does heat enter or leave the system. Carathéodory's formulation of the Second Law of Thermodynamics
Second law of thermodynamics
The second law of thermodynamics is an expression of the tendency that over time, differences in temperature, pressure, and chemical potential equilibrate in an isolated physical system. From the state of thermodynamic equilibrium, the law deduced the principle of the increase of entropy and...
then takes the form: "In the neighbourhood of any initial state, there are states which cannot be approached arbitrarily close through adiabatic changes of state." From this principle he derived the existence of entropy
Entropy
Entropy is a thermodynamic property that can be used to determine the energy available for useful work in a thermodynamic process, such as in energy conversion devices, engines, or machines. Such devices can only be driven by convertible energy, and have a theoretical maximum efficiency when...
as a state function
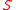
whose differential
 is proportional to the heat differential form
is proportional to the heat differential form 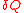 , so it remains constant under adiabatic state changes (in Carathéodory's sense). The increase of entropy during irreversible
, so it remains constant under adiabatic state changes (in Carathéodory's sense). The increase of entropy during irreversibleprocesses is not obvious in this formulation, without further assumptions.
The definition employed by Lieb and Yngvason is rather different since the state changes considered can be the result of arbitrarily complicated, possibly violent, irreversible processes and there is no mention of 'heat' or differential forms. It goes as follows: A state
 is adiabatically accessible from a state
is adiabatically accessible from a state  , in symbols
, in symbols  , if it is possible to transform
, if it is possible to transform  into
into  in such a way that the only net effect of the process on the surroundings is that a weight has been raised or lowered (or a spring is stretched/compressed, or a flywheel is set in motion).
in such a way that the only net effect of the process on the surroundings is that a weight has been raised or lowered (or a spring is stretched/compressed, or a flywheel is set in motion).A definition of thermodynamic entropy can be based entirely on certain properties of the relation
 of adiabatic accessibility that are taken as axioms in the Lieb-Yngvason approach. The entropy has the property that
of adiabatic accessibility that are taken as axioms in the Lieb-Yngvason approach. The entropy has the property that 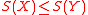 if and only if
if and only if  , in accord with the Second Law.
, in accord with the Second Law.External links
- A. Thess: Was ist Entropie?

