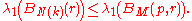In Riemannian geometry

Riemannian geometry

Riemannian geometry is the branch of differential geometry that studies Riemannian manifolds, smooth manifolds with a Riemannian metric, i.e. with an inner product on the tangent space at each point which varies smoothly from point to point. This gives, in particular, local notions of angle, length...

, Cheng's eigenvalue comparison theorem states in general terms that when a domain is large, the first Dirichlet eigenvalue

Dirichlet eigenvalue

In mathematics, the Dirichlet eigenvalues are the fundamental modes of vibration of an idealized drum with a given shape. The problem of whether one can hear the shape of a drum is: given the Dirichlet eigenvalues, what features of the shape of the drum can one deduce. Here a "drum" is thought of...

 of its Laplace–Beltrami operator is small. This general characterization is not precise, in part because the notion of "size" of the domain must also account for its curvature

Curvature

In mathematics, curvature refers to any of a number of loosely related concepts in different areas of geometry. Intuitively, curvature is the amount by which a geometric object deviates from being flat, or straight in the case of a line, but this is defined in different ways depending on the context...

. The theorem is due to . Using geodesic balls, it can be generalized to certain tubular domains .

Theorem

Let M be a Riemannian manifold

Riemannian manifold

In Riemannian geometry and the differential geometry of surfaces, a Riemannian manifold or Riemannian space is a real differentiable manifold M in which each tangent space is equipped with an inner product g, a Riemannian metric, which varies smoothly from point to point...

 with dimension n, and let B_M(p, r) be a geodesic ball centered at p with radius r less than the injectivity radius of p ∈ M. For each real number k, let N(k) denote the simply connected space form

Space form

In mathematics, a space form is a complete Riemannian manifold M of constant sectional curvature K. The three obvious examples are Euclidean n-space, the n-dimensional sphere, and hyperbolic space, although a space form need not be simply connected.-Reduction to generalized crystallography:It is a...

 of dimension n and constant sectional curvature

Sectional curvature

In Riemannian geometry, the sectional curvature is one of the ways to describe the curvature of Riemannian manifolds. The sectional curvature K depends on a two-dimensional plane σp in the tangent space at p...

 k. Cheng's eigenvalue comparison theorem compares the first eigenvalue λ₁(B_M(p, r)) of the Dirichlet problem in B_M(p, r) with the first eigenvalue in B_N(k)(r) for suitable values of k. There are two parts to the theorem:

• Suppose that K_M, the sectional curvature
  Sectional curvature
  In Riemannian geometry, the sectional curvature is one of the ways to describe the curvature of Riemannian manifolds. The sectional curvature K depends on a two-dimensional plane σp in the tangent space at p...
  
   of M, satisfies

Then

The second part is a comparison theorem for the Ricci curvature

Ricci curvature

In differential geometry, the Ricci curvature tensor, named after Gregorio Ricci-Curbastro, represents the amount by which the volume element of a geodesic ball in a curved Riemannian manifold deviates from that of the standard ball in Euclidean space...

 of M:

• Suppose that the Ricci curvature of M satisfies, for every vector field X,

Then, with the same notation as above,

S.Y. Cheng used Barta's theorem to derive the eigenvalue comparison theorem. As a special case, if k = −1 and inj(p) = ∞, Cheng’s inequality becomes λ^*(N) ≥ λ^*(H ⁿ(−1)) which is McKean’s inequality.